28 Mathieu Functions and Hill’s Equation Mathieu Functions of Integer Order 28.1 Special Notation 28.3 Graphics

§28.2 Definitions and Basic Properties

Permalink:: http://dlmf.nist.gov/28.2

§28.2(i) Mathieu’s Equation
§28.2(ii) Basic Solutions $w_{{\mbox{\rm\tiny I}}}$ , $w_{{\mbox{\rm\tiny II}}}$
§28.2(iii) Floquet’s Theorem and the Characteristic Exponents
§28.2(iv) Floquet Solutions
§28.2(v) Eigenvalues $\mathop{a_{{n}}\/}\nolimits$ , $\mathop{b_{{n}}\/}\nolimits$
§28.2(vi) Eigenfunctions

§28.2(i) Mathieu’s Equation

Notes:: See McLachlan (1947, p. 10).
Keywords:: Mathieu functions, Mathieu’s equation, singularities
Referenced by:: §28.12(i)
Permalink:: http://dlmf.nist.gov/28.2.i

The standard form of Mathieu’s equation with parameters is

28.2.1 $w^{{\prime\prime}}+(a-2q\mathop{\cos\/}\nolimits\!\left(2z\right))w=0.$

Symbols:: $\mathop{\cos\/}\nolimits z$ : cosine function, $q=h^{2}$ : parameter, : complex variable, : parameter and : Mathieu’s equation solution
A&S Ref:: 20.3.1 20.1.1 (in slightly different form)
Referenced by:: Figure 28.17.1, Figure 28.17.1, §28.17, §28.17, §28.2(ii), §28.2(ii), §28.2(iii), §28.2(iv), §28.20(i), §28.29(i), §28.32(i), §28.32(i), §28.33(i), §28.33(i), §28.33(iii), §28.34(i), §28.34(ii), §28.34(iii), ¶ ‣ §28.5(i), ¶ ‣ §28.8(iv), ¶ ‣ §28.8(iv), ¶ ‣ §28.8(iv), §30.2(iii)
Permalink:: http://dlmf.nist.gov/28.2.E1
Encodings:: TeX, pMML, png

With $\zeta={\mathop{\sin\/}\nolimits^{{2}}}z$ we obtain the algebraic form of Mathieu’s equation

28.2.2 $\zeta(1-\zeta)w^{{\prime\prime}}+\tfrac{1}{2}\left(1-2\zeta)w^{{\prime}}+% \tfrac{1}{4}(a-2q(1-2\zeta)\right)w=0.$

Symbols:: $q=h^{2}$ : parameter, : parameter, : Mathieu’s equation solution and $\zeta$ : change of variable
Permalink:: http://dlmf.nist.gov/28.2.E2
Encodings:: TeX, pMML, png

This equation has regular singularities at 0 and 1, both with exponents 0 and $\frac{1}{2}$ , and an irregular singular point at $\infty$ . With $\zeta=\mathop{\cos\/}\nolimits z$ we obtain another algebraic form:

28.2.3 $(1-\zeta^{2})w^{{\prime\prime}}-\zeta w^{{\prime}}+\left(a+2q-4q\zeta^{2}% \right)w=0.$

Symbols:: $q=h^{2}$ : parameter, : parameter, : Mathieu’s equation solution and $\zeta$ : change of variable
A&S Ref:: 20.1.7 (in slightly different notation)
Permalink:: http://dlmf.nist.gov/28.2.E3
Encodings:: TeX, pMML, png

§28.2(ii) Basic Solutions $w_{{\mbox{\rm\tiny I}}}$ , $w_{{\mbox{\rm\tiny II}}}$

Notes:: See Arscott (1964b, §2.1.1) and Meixner and Schäfke (1954, §§2.11–2.12).
Keywords:: Mathieu functions, Mathieu’s equation
Referenced by:: §28.12(i), §28.2(vi), §28.29(ii), §28.29(ii), §28.4(vii)
Permalink:: http://dlmf.nist.gov/28.2.ii

Since (28.2.1) has no finite singularities its solutions are entire functions of . Furthermore, a solution with given initial constant values of and $w^{{\prime}}$ at a point $z_{0}$ is an entire function of the three variables , , and .

The following three transformations

28.2.4

$z\to-z;$

$z\to z\pm\pi;$

$z\to z\pm\tfrac{1}{2}\pi,q\to-q;$

Symbols:: $q=h^{2}$ : parameter and : complex variable
Referenced by:: §28.2(vi)
Permalink:: http://dlmf.nist.gov/28.2.E4
Encodings:: TeX, TeX, TeX, pMML, pMML, pMML, png, png, png

each leave (28.2.1) unchanged. (28.2.1) possesses a fundamental pair of solutions $w_{{\mbox{\tiny I}}}(z;a,q),w_{{\mbox{\tiny II}}}(z;a,q)$ called basic solutions with

28.2.5 $\begin{bmatrix}w_{{\mbox{\tiny I}}}(0;a,q)&w_{{\mbox{\tiny II}}}(0;a,q)\\ w^{{\prime}}_{{\mbox{\tiny I}}}(0;a,q)&w^{{\prime}}_{{\mbox{\tiny II}}}(0;a,q)% \end{bmatrix}=\begin{bmatrix}1&0\\ 0&1\end{bmatrix}.$

Symbols:: $q=h^{2}$ : parameter, : parameter, : Mathieu’s equation solution, $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution and $w_{{\mbox{\tiny II}}}(z;a,q)$ : fundamental solution
A&S Ref:: 20.3.9 (in different notation)
Referenced by:: §28.29(ii), §28.34(i), §28.34(ii)
Permalink:: http://dlmf.nist.gov/28.2.E5
Encodings:: TeX, pMML, png

$w_{{\mbox{\tiny I}}}(z;a,q)$ is even and $w_{{\mbox{\tiny II}}}(z;a,q)$ is odd. Other properties are as follows.

28.2.6 $\mathop{\mathscr{W}\/}\nolimits\left\{w_{{\mbox{\tiny I}}},w_{{\mbox{\tiny II}% }}\right\}=1,$

Symbols:: $\mathop{\mathscr{W}\/}\nolimits$ : Wronskian, $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution and $w_{{\mbox{\tiny II}}}(z;a,q)$ : fundamental solution
Referenced by:: §28.29(ii)
Permalink:: http://dlmf.nist.gov/28.2.E6
Encodings:: TeX, pMML, png

28.2.7 $w_{{\mbox{\tiny I}}}(z\pm\pi;a,q)=w_{{\mbox{\tiny I}}}(\pi;a,q)w_{{\mbox{\tiny I% }}}(z;a,q)\pm w^{{\prime}}_{{\mbox{\tiny I}}}(\pi;a,q)w_{{\mbox{\tiny II}}}(z;% a,q),$

Symbols:: $q=h^{2}$ : parameter, : complex variable, : parameter, : Mathieu’s equation solution, $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution and $w_{{\mbox{\tiny II}}}(z;a,q)$ : fundamental solution
Referenced by:: §28.2(iv)
Permalink:: http://dlmf.nist.gov/28.2.E7
Encodings:: TeX, pMML, png

28.2.8 $w_{{\mbox{\tiny II}}}(z\pm\pi;a,q)=\pm w_{{\mbox{\tiny II}}}(\pi;a,q)w_{{\mbox% {\tiny I}}}(z;a,q)+w^{{\prime}}_{{\mbox{\tiny II}}}(\pi;a,q)w_{{\mbox{\tiny II% }}}(z;a,q),$

Symbols:: $q=h^{2}$ : parameter, : complex variable, : parameter, : Mathieu’s equation solution, $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution and $w_{{\mbox{\tiny II}}}(z;a,q)$ : fundamental solution
Permalink:: http://dlmf.nist.gov/28.2.E8
Encodings:: TeX, pMML, png

28.2.9 $w_{{\mbox{\tiny I}}}(\pi;a,q)=w^{{\prime}}_{{\mbox{\tiny II}}}(\pi;a,q),$

Symbols:: $q=h^{2}$ : parameter, : parameter, : Mathieu’s equation solution and $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution
Referenced by:: §28.2(iv)
Permalink:: http://dlmf.nist.gov/28.2.E9
Encodings:: TeX, pMML, png

28.2.10 $w_{{\mbox{\tiny I}}}(\pi;a,q)-1=2w^{{\prime}}_{{\mbox{\tiny I}}}(\tfrac{1}{2}% \pi;a,q)w_{{\mbox{\tiny II}}}(\tfrac{1}{2}\pi;a,q),$

Symbols:: $q=h^{2}$ : parameter, : parameter, : Mathieu’s equation solution, $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution and $w_{{\mbox{\tiny II}}}(z;a,q)$ : fundamental solution
Permalink:: http://dlmf.nist.gov/28.2.E10
Encodings:: TeX, pMML, png

28.2.11 $w_{{\mbox{\tiny I}}}(\pi;a,q)+1=2w_{{\mbox{\tiny I}}}(\tfrac{1}{2}\pi;a,q)w^{{% \prime}}_{{\mbox{\tiny II}}}(\tfrac{1}{2}\pi;a,q),$

Symbols:: $q=h^{2}$ : parameter, : parameter, : Mathieu’s equation solution and $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution
Permalink:: http://dlmf.nist.gov/28.2.E11
Encodings:: TeX, pMML, png

28.2.12 $w^{{\prime}}_{{\mbox{\tiny I}}}(\pi;a,q)=2w_{{\mbox{\tiny I}}}(\tfrac{1}{2}\pi% ;a,q)w^{{\prime}}_{{\mbox{\tiny I}}}(\tfrac{1}{2}\pi;a,q),$

Symbols:: $q=h^{2}$ : parameter, : parameter, : Mathieu’s equation solution and $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution
Permalink:: http://dlmf.nist.gov/28.2.E12
Encodings:: TeX, pMML, png

28.2.13 $w_{{\mbox{\tiny II}}}(\pi;a,q)=2w_{{\mbox{\tiny II}}}(\tfrac{1}{2}\pi;a,q)w^{{% \prime}}_{{\mbox{\tiny II}}}(\tfrac{1}{2}\pi;a,q).$

Symbols:: $q=h^{2}$ : parameter, : parameter, : Mathieu’s equation solution and $w_{{\mbox{\tiny II}}}(z;a,q)$ : fundamental solution
Permalink:: http://dlmf.nist.gov/28.2.E13
Encodings:: TeX, pMML, png

§28.2(iii) Floquet’s Theorem and the Characteristic Exponents

Notes:: See Arscott (1964b, §2.2), Erdélyi et al. (1955, pp. 98–100), and Meixner and Schäfke (1954, §2.13).
Keywords:: Floquet’s theorem, Mathieu’s equation, characteristic equation
Referenced by:: §28.12(i)
Permalink:: http://dlmf.nist.gov/28.2.iii

Let $\nu$ be any real or complex constant. Then Mathieu’s equation (28.2.1) has a nontrivial solution such that

28.2.14 $w(z+\pi)=e^{{\pi i\nu}}w(z),$

Symbols:: : base of exponential function, : complex variable, $\nu$ : complex parameter and : Mathieu’s equation solution
Referenced by:: §28.12(i), §28.2(iv)
Permalink:: http://dlmf.nist.gov/28.2.E14
Encodings:: TeX, pMML, png

iff $e^{{\pi i\nu}}$ is an eigenvalue of the matrix

28.2.15 $\begin{bmatrix}w_{{\mbox{\tiny I}}}(\pi;a,q)&w_{{\mbox{\tiny II}}}(\pi;a,q)\\ w^{{\prime}}_{{\mbox{\tiny I}}}(\pi;a,q)&w^{{\prime}}_{{\mbox{\tiny II}}}(\pi;% a,q)\end{bmatrix}.$

Symbols:: $q=h^{2}$ : parameter, : parameter, : Mathieu’s equation solution, $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution and $w_{{\mbox{\tiny II}}}(z;a,q)$ : fundamental solution
Permalink:: http://dlmf.nist.gov/28.2.E15
Encodings:: TeX, pMML, png

Equivalently,

28.2.16 $\mathop{\cos\/}\nolimits\!\left(\pi\nu\right)=w_{{\mbox{\tiny I}}}(\pi;a,q)=w_% {{\mbox{\tiny I}}}(\pi;a,-q).$

Symbols:: $\mathop{\cos\/}\nolimits z$ : cosine function, $q=h^{2}$ : parameter, $\nu$ : complex parameter, : parameter and $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution
A&S Ref:: 20.3.10 (in slightly different form)
Referenced by:: §28.12(i), §28.12(i), §28.12(ii), §28.2(iii), §28.2(iv), §28.2(v), §28.34(ii), §28.34(i)
Permalink:: http://dlmf.nist.gov/28.2.E16
Encodings:: TeX, pMML, png

This is the characteristic equation of Mathieu’s equation (28.2.1). $\mathop{\cos\/}\nolimits\!\left(\pi\nu\right)$ is an entire function of $a,q^{2}$ . The solutions of (28.2.16) are given by $\nu=\pi^{{-1}}\mathop{\mathrm{arccos}\/}\nolimits\!\left(w_{{\mbox{\tiny I}}}(% \pi;a,q)\right)$ . If the inverse cosine takes its principal value (§4.23(ii)), then $\nu=\widehat{\nu}$ , where $0\leq\realpart{\widehat{\nu}}\leq 1$ . The general solution of (28.2.16) is $\nu=\pm\widehat{\nu}+2n$ , where $n\in\Integer$ . Either $\widehat{\nu}$ or $\nu$ is called a characteristic exponent of (28.2.1). If $\widehat{\nu}=0$ or 1, or equivalently, $\nu=n$ , then $\nu$ is a double root of the characteristic equation, otherwise it is a simple root.

§28.2(iv) Floquet Solutions

Notes:: See Meixner and Schäfke (1954, §2.13 and §2.21). For a proof of the Theorem of Ince see Arscott (1964b, §2.4).
Keywords:: Floquet solutions, Fourier series, Fourier-series expansions, Ince’s theorem, Mathieu functions, Mathieu’s equation, Theorem of Ince, pseudoperiodic solutions
Permalink:: http://dlmf.nist.gov/28.2.iv

A solution with the pseudoperiodic property (28.2.14) is called a Floquet solution with respect to $\nu$ . (28.2.9), (28.2.16), and (28.2.7) give for each solution of (28.2.1) the connection formula

28.2.17 $w(z+\pi)+w(z-\pi)=2\mathop{\cos\/}\nolimits\!\left(\pi\nu\right)w(z).$

Symbols:: $\mathop{\cos\/}\nolimits z$ : cosine function, : complex variable, $\nu$ : complex parameter and : Mathieu’s equation solution
Permalink:: http://dlmf.nist.gov/28.2.E17
Encodings:: TeX, pMML, png

Therefore a nontrivial solution is either a Floquet solution with respect to $\nu$ , or $w(z+\pi)-e^{{i\nu\pi}}w(z)$ is a Floquet solution with respect to $-\nu$ .

If $q\neq 0$ , then for a given value of $\nu$ the corresponding Floquet solution is unique, except for an arbitrary constant factor (Theorem of Ince; see also 28.5(i)).

The Fourier series of a Floquet solution

28.2.18 $w(z)=\sum_{{n=-\infty}}^{{\infty}}c_{{2n}}e^{{i(\nu+2n)z}}$

Symbols:: : base of exponential function, : integer, : complex variable, $\nu$ : complex parameter, : Mathieu’s equation solution and $c_{{2n}}$ : coefficients
A&S Ref:: 20.3.8
Permalink:: http://dlmf.nist.gov/28.2.E18
Encodings:: TeX, pMML, png

converges absolutely and uniformly in compact subsets of $\Complex$ . The coefficients $c_{{2n}}$ satisfy

28.2.19 ${qc_{{2n+2}}-\left(a-(\nu+2n)^{2}\right)c_{{2n}}+qc_{{2n-2}}=0,}$ $n\in\Integer$ .

Symbols:: $\in$ : element of, $\Integer$ : set of all integers, $q=h^{2}$ : parameter, : integer, $\nu$ : complex parameter, : parameter and $c_{{2n}}$ : coefficients
A&S Ref:: 20.3.12 20.3.13
Referenced by:: §28.2(iv)
Permalink:: http://dlmf.nist.gov/28.2.E19
Encodings:: TeX, pMML, png

Conversely, a nontrivial solution $c_{{2n}}$ of (28.2.19) that satisfies

28.2.20 $\lim_{{n\to\pm\infty}}|c_{{2n}}|^{{1/|n|}}=0$

Symbols:: : integer and $c_{{2n}}$ : coefficients
Permalink:: http://dlmf.nist.gov/28.2.E20
Encodings:: TeX, pMML, png

leads to a Floquet solution.

§28.2(v) Eigenvalues $\mathop{a_{{n}}\/}\nolimits$ , $\mathop{b_{{n}}\/}\nolimits$

Notes:: See Erdélyi et al. (1955, §16.4), Meixner and Schäfke (1954, pp. 108–109), and Meixner et al. (1980, p. 88). Figure 28.2.1 was produced at NIST.
Defines:: $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation and $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation
Keywords:: Mathieu’s equation
Referenced by:: §28.31(ii), §28.34(ii)
Permalink:: http://dlmf.nist.gov/28.2.v

For given $\nu$ and , equation (28.2.16) determines an infinite discrete set of values of , the eigenvalues or characteristic values, of Mathieu’s equation. When $\widehat{\nu}=0$ or 1, the notation for the two sets of eigenvalues corresponding to each $\widehat{\nu}$ is shown in Table 28.2.1, together with the boundary conditions of the associated eigenvalue problem. In Table 28.2.1 $n=0,1,2,\dots$ .

Table 28.2.1: Eigenvalues of Mathieu’s equation.

$\widehat{\nu}$	Boundary Conditions	Eigenvalues
0	$w^{{\prime}}(0)=w^{{\prime}}(\tfrac{1}{2}\pi)=0$	$\mathop{a_{{2n}}\/}\nolimits\!\left(q\right)$
1	$w^{{\prime}}(0)=w(\tfrac{1}{2}\pi)=0$	$\mathop{a_{{2n+1}}\/}\nolimits\!\left(q\right)$
1	$w(0)=w^{{\prime}}(\tfrac{1}{2}\pi)=0$	$\mathop{b_{{2n+1}}\/}\nolimits\!\left(q\right)$
0	$w(0)=w(\tfrac{1}{2}\pi)=0$	$\mathop{b_{{2n+2}}\/}\nolimits\!\left(q\right)$

Symbols:: $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $q=h^{2}$ : parameter, : integer, $\nu$ : complex parameter and : Mathieu’s equation solution
Referenced by:: §28.2(v), §28.2(vi)
Permalink:: http://dlmf.nist.gov/28.2.T1

An equivalent formulation is given by

28.2.21 $\begin{array}[]{ll}w^{{\prime}}_{{\mbox{\tiny I}}}(\tfrac{1}{2}\pi;a,q)=0,&a=% \mathop{a_{{2n}}\/}\nolimits\!\left(q\right),\\ w_{{\mbox{\tiny I}}}(\tfrac{1}{2}\pi;a,q)=0,&a=\mathop{a_{{2n+1}}\/}\nolimits% \!\left(q\right),\end{array}$

Symbols:: $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $q=h^{2}$ : parameter, : integer, : parameter, : Mathieu’s equation solution and $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution
Referenced by:: §28.2(vi), §28.29(ii), §28.7
Permalink:: http://dlmf.nist.gov/28.2.E21
Encodings:: TeX, pMML, png

and

28.2.22 $\begin{array}[]{ll}w^{{\prime}}_{{\mbox{\tiny II}}}(\tfrac{1}{2}\pi;a,q)=0,&a=% \mathop{b_{{2n+1}}\/}\nolimits\!\left(q\right),\\ w_{{\mbox{\tiny II}}}(\tfrac{1}{2}\pi;a,q)=0,&a=\mathop{b_{{2n+2}}\/}\nolimits% \!\left(q\right),\end{array}$

Symbols:: $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $q=h^{2}$ : parameter, : integer, : parameter, : Mathieu’s equation solution and $w_{{\mbox{\tiny II}}}(z;a,q)$ : fundamental solution
Referenced by:: §28.2(vi), §28.29(ii), §28.7
Permalink:: http://dlmf.nist.gov/28.2.E22
Encodings:: TeX, pMML, png

where $n=0,1,2,\dots$ . When ,

28.2.23 $\mathop{a_{{n}}\/}\nolimits\!\left(0\right)=n^{2},$ $n=0,1,2,\dots$ ,

Symbols:: $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation and : integer
Permalink:: http://dlmf.nist.gov/28.2.E23
Encodings:: TeX, pMML, png

28.2.24 $\mathop{b_{{n}}\/}\nolimits\!\left(0\right)=n^{2},$ $n=1,2,3,\dots$ .

Symbols:: $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation and : integer
Permalink:: http://dlmf.nist.gov/28.2.E24
Encodings:: TeX, pMML, png

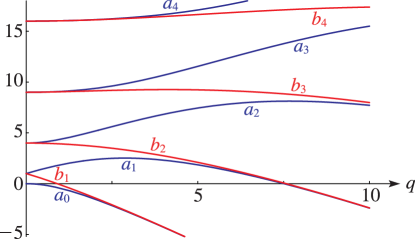
Near , $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ and $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ can be expanded in power series in (see §28.6(i)); elsewhere they are determined by analytic continuation (see §28.7). For nonnegative real values of , see Figure 28.2.1.

Figure 28.2.1: Eigenvalues $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ , $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ of Mathieu’s equation as functions of

for $0\leq q\leq 10$ ,

(

’s),

(

’s).

Symbols:: $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $q=h^{2}$ : parameter, : integer and : parameter
A&S Ref:: 20.1 (Figure)
Referenced by:: Figure 28.13.1, Figure 28.13.1, §28.17, §28.2(v), §28.2(v), §28.8(i)
Permalink:: http://dlmf.nist.gov/28.2.F1
Encodings:: pdf, png

¶ Distribution

Keywords:: Mathieu’s equation

28.2.25 $\begin{array}[]{rl}\mbox{for $q>0$:}&\mathop{a_{{0}}\/}\nolimits<\mathop{b_{{1% }}\/}\nolimits<\mathop{a_{{1}}\/}\nolimits<\mathop{b_{{2}}\/}\nolimits<\mathop% {a_{{2}}\/}\nolimits<\mathop{b_{{3}}\/}\nolimits<\cdots,\\ \mbox{for $q<0$:}&\mathop{a_{{0}}\/}\nolimits<\mathop{a_{{1}}\/}\nolimits<% \mathop{b_{{1}}\/}\nolimits<\mathop{b_{{2}}\/}\nolimits<\mathop{a_{{2}}\/}% \nolimits<\mathop{a_{{3}}\/}\nolimits<\cdots.\end{array}$

Symbols:: $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation and $q=h^{2}$ : parameter
Permalink:: http://dlmf.nist.gov/28.2.E25
Encodings:: TeX, pMML, png

¶ Change of Sign of

Keywords:: Mathieu’s equation

28.2.26 $\mathop{a_{{2n}}\/}\nolimits\!\left(-q\right)=\mathop{a_{{2n}}\/}\nolimits\!% \left(q\right),$

Symbols:: $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $q=h^{2}$ : parameter and : integer
A&S Ref:: 20.8.3
Permalink:: http://dlmf.nist.gov/28.2.E26
Encodings:: TeX, pMML, png

28.2.27 $\mathop{a_{{2n+1}}\/}\nolimits\!\left(-q\right)=\mathop{b_{{2n+1}}\/}\nolimits% \!\left(q\right),$

Symbols:: $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $q=h^{2}$ : parameter and : integer
A&S Ref:: 20.8.3
Permalink:: http://dlmf.nist.gov/28.2.E27
Encodings:: TeX, pMML, png

28.2.28 $\mathop{b_{{2n+2}}\/}\nolimits\!\left(-q\right)=\mathop{b_{{2n+2}}\/}\nolimits% \!\left(q\right).$

Symbols:: $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $q=h^{2}$ : parameter and : integer
A&S Ref:: 20.8.3
Permalink:: http://dlmf.nist.gov/28.2.E28
Encodings:: TeX, pMML, png

§28.2(vi) Eigenfunctions

Notes:: See Meixner and Schäfke (1954, §2.71).
Defines:: $\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function and $\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function
A&S Ref:: 20.8.4
Keywords:: Mathieu functions, Mathieu’s equation
Referenced by:: §28.12(iii), §28.31(ii), ¶ ‣ §28.5(i), §29.5
Permalink:: http://dlmf.nist.gov/28.2.vi

Table 28.2.2 gives the notation for the eigenfunctions corresponding to the eigenvalues in Table 28.2.1. Period $\pi$ means that the eigenfunction has the property $w(z+\pi)=w(z)$ , whereas antiperiod $\pi$ means that $w(z+\pi)=-w(z)$ . Even parity means , and odd parity means .

Table 28.2.2: Eigenfunctions of Mathieu’s equation.

Eigenvalues	Eigenfunctions	Periodicity	Parity
$\mathop{a_{{2n}}\/}\nolimits\!\left(q\right)$	$\mathop{\mathrm{ce}_{{2n}}\/}\nolimits\!\left(z,q\right)$	Period $\pi$	Even
$\mathop{a_{{2n+1}}\/}\nolimits\!\left(q\right)$	$\mathop{\mathrm{ce}_{{2n+1}}\/}\nolimits\!\left(z,q\right)$	Antiperiod $\pi$	Even
$\mathop{b_{{2n+1}}\/}\nolimits\!\left(q\right)$	$\mathop{\mathrm{se}_{{2n+1}}\/}\nolimits\!\left(z,q\right)$	Antiperiod $\pi$	Odd
$\mathop{b_{{2n+2}}\/}\nolimits\!\left(q\right)$	$\mathop{\mathrm{se}_{{2n+2}}\/}\nolimits\!\left(z,q\right)$	Period $\pi$	Odd

Symbols:: $\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $q=h^{2}$ : parameter, : integer and : complex variable
Referenced by:: §28.2(vi)
Permalink:: http://dlmf.nist.gov/28.2.T2

When ,

28.2.29

$\mathop{\mathrm{ce}_{{0}}\/}\nolimits\!\left(z,0\right)=1/\sqrt{2},$

$\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,0\right)=\mathop{\cos\/}% \nolimits\!\left(nz\right),$

$\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,0\right)=\mathop{\sin\/}% \nolimits\!\left(nz\right)$ , $n=1,2,3,\dots$ .

Symbols:: $\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $\mathop{\cos\/}\nolimits z$ : cosine function, $\mathop{\sin\/}\nolimits z$ : sine function, : integer and : complex variable
Referenced by:: §28.2(vi), ¶ ‣ §28.5(i)
Permalink:: http://dlmf.nist.gov/28.2.E29
Encodings:: TeX, TeX, TeX, pMML, pMML, pMML, png, png, png

For simple roots of the corresponding equations (28.2.21) and (28.2.22), the functions are made unique by the normalizations

28.2.30

$\int_{0}^{{2\pi}}\left(\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(x,q\right)% \right)^{2}dx=\pi,$

$\int_{0}^{{2\pi}}\left(\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(x,q\right)% \right)^{2}dx=\pi,$

Symbols:: $\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, : differential of , $\int$ : integral, $q=h^{2}$ : parameter, : integer and : real variable
A&S Ref:: 20.5.3
Permalink:: http://dlmf.nist.gov/28.2.E30
Encodings:: TeX, TeX, pMML, pMML, png, png

the ambiguity of sign being resolved by (28.2.29) when and by continuity for the other values of .

The functions are orthogonal, that is,

28.2.31 $\int_{0}^{{2\pi}}\mathop{\mathrm{ce}_{{m}}\/}\nolimits\!\left(x,q\right)% \mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(x,q\right)dx=0,$ $n\neq m$ ,

Symbols:: $\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, : differential of , $\int$ : integral, : integer, $q=h^{2}$ : parameter, : integer and : real variable
Permalink:: http://dlmf.nist.gov/28.2.E31
Encodings:: TeX, pMML, png

28.2.32 $\int_{0}^{{2\pi}}\mathop{\mathrm{se}_{{m}}\/}\nolimits\!\left(x,q\right)% \mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(x,q\right)dx=0,$ $n\neq m$ ,

Symbols:: $\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, : differential of , $\int$ : integral, : integer, $q=h^{2}$ : parameter, : integer and : real variable
Permalink:: http://dlmf.nist.gov/28.2.E32
Encodings:: TeX, pMML, png

28.2.33 $\int_{0}^{{2\pi}}\mathop{\mathrm{ce}_{{m}}\/}\nolimits\!\left(x,q\right)% \mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(x,q\right)dx=0.$

Symbols:: $\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, : differential of , $\int$ : integral, : integer, $q=h^{2}$ : parameter, : integer and : real variable
Permalink:: http://dlmf.nist.gov/28.2.E33
Encodings:: TeX, pMML, png

For change of sign of (compare (28.2.4))

28.2.34 $\mathop{\mathrm{ce}_{{2n}}\/}\nolimits\!\left(z,-q\right)=(-1)^{n}\mathop{% \mathrm{ce}_{{2n}}\/}\nolimits\!\left(\tfrac{1}{2}\pi-z,q\right),$

Symbols:: $\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $q=h^{2}$ : parameter, : integer and : complex variable
Permalink:: http://dlmf.nist.gov/28.2.E34
Encodings:: TeX, pMML, png

28.2.35 $\mathop{\mathrm{ce}_{{2n+1}}\/}\nolimits\!\left(z,-q\right)=(-1)^{n}\mathop{% \mathrm{se}_{{2n+1}}\/}\nolimits\!\left(\tfrac{1}{2}\pi-z,q\right),$

Symbols:: $\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $q=h^{2}$ : parameter, : integer and : complex variable
Permalink:: http://dlmf.nist.gov/28.2.E35
Encodings:: TeX, pMML, png

28.2.36 $\mathop{\mathrm{se}_{{2n+1}}\/}\nolimits\!\left(z,-q\right)=(-1)^{n}\mathop{% \mathrm{ce}_{{2n+1}}\/}\nolimits\!\left(\tfrac{1}{2}\pi-z,q\right),$

Symbols:: $\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $q=h^{2}$ : parameter, : integer and : complex variable
Permalink:: http://dlmf.nist.gov/28.2.E36
Encodings:: TeX, pMML, png

28.2.37 $\mathop{\mathrm{se}_{{2n+2}}\/}\nolimits\!\left(z,-q\right)=(-1)^{n}\mathop{% \mathrm{se}_{{2n+2}}\/}\nolimits\!\left(\tfrac{1}{2}\pi-z,q\right).$

Symbols:: $\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $q=h^{2}$ : parameter, : integer and : complex variable
Permalink:: http://dlmf.nist.gov/28.2.E37
Encodings:: TeX, pMML, png

For the connection with the basic solutions in §28.2(ii),

28.2.38 $\frac{\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)}{\mathop{\mathrm% {ce}_{{n}}\/}\nolimits\!\left(0,q\right)}=w_{{\mbox{\tiny I}}}(z;\mathop{a_{{n% }}\/}\nolimits\!\left(q\right),q),$ $n=0,1,\dots$ ,

Symbols:: $\mathop{\mathrm{ce}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $\mathop{a_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $q=h^{2}$ : parameter, : integer, : complex variable and $w_{{\mbox{\tiny I}}}(z;a,q)$ : fundamental solution
Permalink:: http://dlmf.nist.gov/28.2.E38
Encodings:: TeX, pMML, png

28.2.39 $\frac{\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)}{{\mathop{% \mathrm{se}_{{n}}\/}\nolimits^{{\prime}}}\!\left(0,q\right)}=w_{{\mbox{\tiny II% }}}(z;\mathop{b_{{n}}\/}\nolimits\!\left(q\right),q),$ $n=1,2,\dots$ .

Symbols:: $\mathop{b_{{n}}\/}\nolimits\!\left(q\right)$ : eigenvalues of Mathieu equation, $\mathop{\mathrm{se}_{{n}}\/}\nolimits\!\left(z,q\right)$ : Mathieu function, $q=h^{2}$ : parameter, : integer, : complex variable and $w_{{\mbox{\tiny II}}}(z;a,q)$ : fundamental solution
Permalink:: http://dlmf.nist.gov/28.2.E39
Encodings:: TeX, pMML, png