§22.4 Periods, Poles, and Zeros

Permalink:: http://dlmf.nist.gov/22.4

§22.4(i) Distribution
§22.4(ii) Graphical Interpretation via Glaisher’s Notation
§22.4(iii) Translation by Half or Quarter Periods

§22.4(i) Distribution

Notes:: See Lawden (1989, pp. 24–30), Walker (1996, pp. 126–131), Whittaker and Watson (1927, pp. 491–498).
Keywords:: Jacobian elliptic functions
Referenced by:: Version 1.0.3 (Aug 29, 2011)
Permalink:: http://dlmf.nist.gov/22.4.i
Addition (effective with 1.0.3):: The paragraph on the distribution of the $k$ -zeros was added.
Reported 2011-08-21

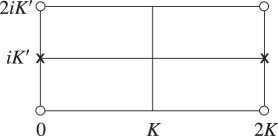
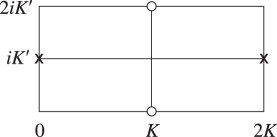
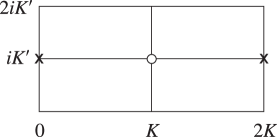
For each Jacobian function, Table 22.4.1 gives its periods in the $z$ -plane in the left column, and the position of one of its poles in the second row. The other poles are at congruent points, which is the set of points obtained by making translations by $2mK+2niK^{{\prime}}$ , where $m,n\in\Integer$ . For example, the poles of $\mathop{\mathrm{sn}\/}\nolimits\left(z,k\right)$ , abbreviated as $\mathop{\mathrm{sn}\/}\nolimits$ in the following tables, are at $z=2mK+(2n+1)iK^{{\prime}}$ .

Table 22.4.1: Periods and poles of Jacobian elliptic functions.

Periods	$z$ -Poles
Periods	$iK^{{\prime}}$	$K+iK^{{\prime}}$	$K$	0
$4K$ , $2iK^{{\prime}}$	$\mathop{\mathrm{sn}\/}\nolimits$	$\mathop{\mathrm{cd}\/}\nolimits$	$\mathop{\mathrm{dc}\/}\nolimits$	$\mathop{\mathrm{ns}\/}\nolimits$
$4K$ , $2K+2iK^{{\prime}}$	$\mathop{\mathrm{cn}\/}\nolimits$	$\mathop{\mathrm{sd}\/}\nolimits$	$\mathop{\mathrm{nc}\/}\nolimits$	$\mathop{\mathrm{ds}\/}\nolimits$
$2K$ , $4iK^{{\prime}}$	$\mathop{\mathrm{dn}\/}\nolimits$	$\mathop{\mathrm{nd}\/}\nolimits$	$\mathop{\mathrm{sc}\/}\nolimits$	$\mathop{\mathrm{cs}\/}\nolimits$

Three functions in the same column of Table 22.4.1 are copolar, and four functions in the same row are coperiodic.

Table 22.4.2 displays the periods and zeros of the functions in the $z$ -plane in a similar manner to Table 22.4.1. Again, one member of each congruent set of zeros appears in the second row; all others are generated by translations of the form $2mK+2niK^{{\prime}}$ , where $m,n\in\Integer$ .

Table 22.4.2: Periods and zeros of Jacobian elliptic functions.

Periods	$z$ -Zeros
Periods	0	$K$	$K+iK^{{\prime}}$	$iK^{{\prime}}$
$4K$ , $2iK^{{\prime}}$	$\mathop{\mathrm{sn}\/}\nolimits$	$\mathop{\mathrm{cd}\/}\nolimits$	$\mathop{\mathrm{dc}\/}\nolimits$	$\mathop{\mathrm{ns}\/}\nolimits$
$4K$ , $2K+2iK^{{\prime}}$	$\mathop{\mathrm{sd}\/}\nolimits$	$\mathop{\mathrm{cn}\/}\nolimits$	$\mathop{\mathrm{ds}\/}\nolimits$	$\mathop{\mathrm{nc}\/}\nolimits$
$2K$ , $4iK^{{\prime}}$	$\mathop{\mathrm{sc}\/}\nolimits$	$\mathop{\mathrm{cs}\/}\nolimits$	$\mathop{\mathrm{dn}\/}\nolimits$	$\mathop{\mathrm{nd}\/}\nolimits$

Symbols:: $\mathop{\mathrm{cd}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{cn}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{cs}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{dc}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{dn}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{ds}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{nc}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{nd}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{ns}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{sc}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{sd}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{sn}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{{K^{{\prime}}}\/}\nolimits\!\left(k\right)$ : Legendre’s complementary complete elliptic integral of the first kind, $\mathop{K\/}\nolimits\!\left(k\right)$ : Legendre’s complete elliptic integral of the first kind and $z$ : complex
A&S Ref:: Table 16.2 (modified)
Referenced by:: §22.4(i)
Permalink:: http://dlmf.nist.gov/22.4.T2

Figure 22.4.1 illustrates the locations in the $z$ -plane of the poles and zeros of the three principal Jacobian functions in the rectangle with vertices 0, $2K$ , $2K+2iK^{{\prime}}$ , $2iK^{{\prime}}$ . The other poles and zeros are at the congruent points.


(a) $\mathop{\mathrm{sn}\/}\nolimits\left(z,k\right)$	(b) $\mathop{\mathrm{cn}\/}\nolimits\left(z,k\right)$	(c) $\mathop{\mathrm{dn}\/}\nolimits\left(z,k\right)$

Figure 22.4.1: $z$ -plane. Poles $\boldsymbol{\times\times\times}$ and zeros $\boldsymbol{\circ\circ\circ}$ of the principal Jacobian elliptic functions.

Symbols:: $\mathop{\mathrm{cn}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{dn}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $\mathop{\mathrm{sn}\/}\nolimits\left(z,k\right)$ : Jacobian elliptic function, $z$ : complex and $k$ : modulus
Referenced by:: §22.4(i)
Permalink:: http://dlmf.nist.gov/22.4.F1
Encodings:: pdf, pdf, pdf, png, png, png

For the distribution of the $k$ -zeros of the Jacobian elliptic functions see Walker (2009).

§22.4(ii) Graphical Interpretation via Glaisher’s Notation

Notes:: See Walker (1996, pp. 126–131), Whittaker and Watson (1927, pp. 498–505).
Keywords:: Glaisher’s notation, Jacobian elliptic functions, lattice
Permalink:: http://dlmf.nist.gov/22.4.ii

Figure 22.4.2 depicts the fundamental unit cell in the $z$ -plane, with vertices $\mbox{s}=0$ , $\mbox{c}=K$ , $\mbox{d}=K+iK^{{\prime}}$ , $\mbox{n}=iK^{{\prime}}$ . The set of points $z=mK+niK^{{\prime}}$ , $m,n\in\Integer$ , comprise the lattice for the 12 Jacobian functions; all other lattice unit cells are generated by translation of the fundamental unit cell by $mK+niK^{{\prime}}$ , where again $m,n\in\Integer$ .

Figure 22.4.2: $z$ -plane. Fundamental unit cell.

Symbols:: $z$ : complex
Referenced by:: §22.4(ii), §22.4(ii)
Permalink:: http://dlmf.nist.gov/22.4.F2
Encodings:: pdf, png

Using the p,q notation of (22.2.10), Figure 22.4.2 serves as a mnemonic for the poles, zeros, periods, and half-periods of the 12 Jacobian elliptic functions as follows. Let p,q be any two distinct letters from the set s,c,d,n which appear in counterclockwise orientation at the corners of all lattice unit cells. Then: (a) In any lattice unit cell $\mathop{\mathrm{pq}\/}\nolimits\left(z,k\right)$ has a simple zero at $z=\mbox{p}$ and a simple pole at $z=\mbox{q}$ . (b) The difference between p and the nearest q is a half-period of $\mathop{\mathrm{pq}\/}\nolimits\left(z,k\right)$ . This half-period will be plus or minus a member of the triple ${K,iK^{{\prime}},K+iK^{{\prime}}}$ ; the other two members of this triple are quarter periods of $\mathop{\mathrm{pq}\/}\nolimits\left(z,k\right)$ .

§22.4(iii) Translation by Half or Quarter Periods

Notes:: See Lawden (1989, pp. 26–30), Whittaker and Watson (1927, pp. 498–505).
Keywords:: Jacobian elliptic functions
Referenced by:: §23.6(ii)
Permalink:: http://dlmf.nist.gov/22.4.iii

See Table 22.4.3.

For example, $\mathop{\mathrm{sn}\/}\nolimits\left(z+K,k\right)=\mathop{\mathrm{cd}\/}\nolimits\left(z,k\right)$ . (The modulus $k$ is suppressed throughout the table.)

Table 22.4.3: Half- or quarter-period shifts of variable for the Jacobian elliptic functions.

	$u$
	$z+K$	$z+K+iK^{{\prime}}$	$z+iK^{{\prime}}$	$z+2K$	$z+2K+2iK^{{\prime}}$	$z+2iK^{{\prime}}$
$\mathop{\mathrm{sn}\/}\nolimits u$	$\mathop{\mathrm{cd}\/}\nolimits z$	$k^{{-1}}\mathop{\mathrm{dc}\/}\nolimits z$	$k^{{-1}}\mathop{\mathrm{ns}\/}\nolimits z$	$-\mathop{\mathrm{sn}\/}\nolimits z$	$-\mathop{\mathrm{sn}\/}\nolimits z$	$\mathop{\mathrm{sn}\/}\nolimits z$
$\mathop{\mathrm{cn}\/}\nolimits u$	$-k^{{\prime}}\mathop{\mathrm{sd}\/}\nolimits z$	$-ik^{{\prime}}k^{{-1}}\mathop{\mathrm{nc}\/}\nolimits z$	$-ik^{{-1}}\mathop{\mathrm{ds}\/}\nolimits z$	$-\mathop{\mathrm{cn}\/}\nolimits z$	$\mathop{\mathrm{cn}\/}\nolimits z$	$-\mathop{\mathrm{cn}\/}\nolimits z$
$\mathop{\mathrm{dn}\/}\nolimits u$	$k^{{\prime}}\mathop{\mathrm{nd}\/}\nolimits z$	$ik^{{\prime}}\mathop{\mathrm{sc}\/}\nolimits z$	$-i\mathop{\mathrm{cs}\/}\nolimits z$	$\mathop{\mathrm{dn}\/}\nolimits z$	$-\mathop{\mathrm{dn}\/}\nolimits z$	$-\mathop{\mathrm{dn}\/}\nolimits z$
$\mathop{\mathrm{cd}\/}\nolimits u$	$-\mathop{\mathrm{sn}\/}\nolimits z$	$k^{{-1}}\mathop{\mathrm{ns}\/}\nolimits z$	$k^{{-1}}\mathop{\mathrm{dc}\/}\nolimits z$	$-\mathop{\mathrm{cd}\/}\nolimits z$	$-\mathop{\mathrm{cd}\/}\nolimits z$	$\mathop{\mathrm{cd}\/}\nolimits z$
$\mathop{\mathrm{sd}\/}\nolimits u$	${k^{{\prime}}}^{{-1}}\mathop{\mathrm{cn}\/}\nolimits z$	$-i(kk^{{\prime}})^{{-1}}\mathop{\mathrm{ds}\/}\nolimits z$	$ik^{{-1}}\mathop{\mathrm{nc}\/}\nolimits z$	$-\mathop{\mathrm{sd}\/}\nolimits z$	$\mathop{\mathrm{sd}\/}\nolimits z$	$-\mathop{\mathrm{sd}\/}\nolimits z$
$\mathop{\mathrm{nd}\/}\nolimits u$	${k^{{\prime}}}^{{-1}}\mathop{\mathrm{dn}\/}\nolimits z$	$-i{k^{{\prime}}}^{{-1}}\mathop{\mathrm{cs}\/}\nolimits z$	$i\mathop{\mathrm{sc}\/}\nolimits z$	$\mathop{\mathrm{nd}\/}\nolimits z$	$-\mathop{\mathrm{nd}\/}\nolimits z$	$-\mathop{\mathrm{nd}\/}\nolimits z$
$\mathop{\mathrm{dc}\/}\nolimits u$	$-\mathop{\mathrm{ns}\/}\nolimits z$	$k\mathop{\mathrm{sn}\/}\nolimits z$	$k\mathop{\mathrm{cd}\/}\nolimits z$	$-\mathop{\mathrm{dc}\/}\nolimits z$	$-\mathop{\mathrm{dc}\/}\nolimits z$	$\mathop{\mathrm{dc}\/}\nolimits z$
$\mathop{\mathrm{nc}\/}\nolimits u$	$-{k^{{\prime}}}^{{-1}}\mathop{\mathrm{ds}\/}\nolimits z$	$ik{k^{{\prime}}}^{{-1}}\mathop{\mathrm{cn}\/}\nolimits z$	$ik\mathop{\mathrm{sd}\/}\nolimits z$	$-\mathop{\mathrm{nc}\/}\nolimits z$	$\mathop{\mathrm{nc}\/}\nolimits z$	$-\mathop{\mathrm{nc}\/}\nolimits z$
$\mathop{\mathrm{sc}\/}\nolimits u$	$-{k^{{\prime}}}^{{-1}}\mathop{\mathrm{cs}\/}\nolimits z$	$i{k^{{\prime}}}^{{-1}}\mathop{\mathrm{dn}\/}\nolimits z$	$i\mathop{\mathrm{nd}\/}\nolimits z$	$\mathop{\mathrm{sc}\/}\nolimits z$	$-\mathop{\mathrm{sc}\/}\nolimits z$	$-\mathop{\mathrm{sc}\/}\nolimits z$
$\mathop{\mathrm{ns}\/}\nolimits u$	$\mathop{\mathrm{dc}\/}\nolimits z$	$k\mathop{\mathrm{cd}\/}\nolimits z$	$k\mathop{\mathrm{sn}\/}\nolimits z$	$-\mathop{\mathrm{ns}\/}\nolimits z$	$-\mathop{\mathrm{ns}\/}\nolimits z$	$\mathop{\mathrm{ns}\/}\nolimits z$
$\mathop{\mathrm{ds}\/}\nolimits u$	$k^{{\prime}}\mathop{\mathrm{nc}\/}\nolimits z$	$ikk^{{\prime}}\mathop{\mathrm{sd}\/}\nolimits z$	$-ik\mathop{\mathrm{cn}\/}\nolimits z$	$-\mathop{\mathrm{ds}\/}\nolimits z$	$\mathop{\mathrm{ds}\/}\nolimits z$	$-\mathop{\mathrm{ds}\/}\nolimits z$
$\mathop{\mathrm{cs}\/}\nolimits u$	$-k^{{\prime}}\mathop{\mathrm{sc}\/}\nolimits z$	$-ik^{{\prime}}\mathop{\mathrm{nd}\/}\nolimits z$	$-i\mathop{\mathrm{dn}\/}\nolimits z$	$\mathop{\mathrm{cs}\/}\nolimits z$	$-\mathop{\mathrm{cs}\/}\nolimits z$	$-\mathop{\mathrm{cs}\/}\nolimits z$

§22.4 Periods, Poles, and Zeros

Contents

§22.4(i) Distribution

§22.4(ii) Graphical Interpretation via Glaisher’s Notation

§22.4(iii) Translation by Half or Quarter Periods