Jacobi%20theta%20functions

SearchAdvancedHelp

(0.006 seconds)

1—10 of 12 matching pages

1: 20.11 Generalizations and Analogs

§20.11 Generalizations and Analogs

… ►

§20.11(ii) Ramanujan’s Theta Function and $q$-Series

… ► … ►

§20.11(iv) Theta Functions with Characteristics

… ►For $m=1,2,3,4$, $n=1,2,3,4$, and $m\ne n$, define twelve combined theta functions ${\phi }_{m,n}(z,q)$ by …

2: 20.7 Identities

… ►

20.7.34 $$\frac{{\theta }_1(z,q^2){\theta }_3(z,q^2)}{{\theta }_1(z,\mathrm{i}q)}=\frac{{\theta }_2(z,q^2){\theta }_4(z,q^2)}{{\theta }_2(z,\mathrm{i}q)}={\mathrm{i}}^{-1/4}\sqrt{\frac{{\theta }_2(0,q^2){\theta }_4(0,q^2)}{2}}.$$

ⓘ

Symbols:

${\theta }_j(z,q)$: theta function, $\mathrm{i}$: imaginary unit, $z$: complex and $q$: nome

Referenced by:

§20.7(iv), Erratum (V1.0.5) for Chapters 8, 20, 36

Permalink:

http://dlmf.nist.gov/20.7.E34

Encodings:

pMML, png, TeX

Addition (effective with 1.0.5):

This equation has been added. See Walker (2012).

See also:

Annotations for §20.7(ix), §20.7 and Ch.20

…

3: 20.10 Integrals

§20.10 Integrals

►

§20.10(i) Mellin Transforms with respect to the Lattice Parameter

… ►

§20.10(ii) Laplace Transforms with respect to the Lattice Parameter

… ►For corresponding results for argument derivatives of the theta functions see Erdélyi et al. (1954a, pp. 224–225) or Oberhettinger and Badii (1973, p. 193). … ►For further integrals of theta functions see Erdélyi et al. (1954a, pp. 61–62 and 339), Prudnikov et al. (1990, pp. 356–358), Prudnikov et al. (1992a, §3.41), and Gradshteyn and Ryzhik (2000, pp. 627–628).

4: Bibliography B

… ►

The generating function of Jacobi polynomials. J. London Math. Soc. 13, pp. 8–12.

ⓘ

External Links:

ISSN 0024-6107; 1469-7750/e, Document, ZentralBlatt

Referenced by:

§18.18(vii).

Encodings:

BibTeX

… ►

A program for computing the Riemann zeta function for complex argument. Comput. Phys. Comm. 20 (3), pp. 441–445.

ⓘ

Notes:

Double-precision Fortran, maximum accuracy 10S.

External Links:

Document, Code, ZentralBlatt

Referenced by:

1st item.

Encodings:

BibTeX

… ►

Coulomb functions (negative energies). Comput. Phys. Comm. 20 (3), pp. 447–458.

ⓘ

Notes:

Double-precision Fortran code.

External Links:

Document, Code

Referenced by:

1st item, 4th item.

Encodings:

BibTeX

… ►

A short table of the functions ${\mathrm{Ki}}_n(x)$, from $n=1$ to $n=16$ . Phil. Mag. Series 7 20, pp. 343–347.

ⓘ

External Links:

Document, ZentralBlatt

Referenced by:

§10.43(iii), 2nd item.

Encodings:

BibTeX

… ►

Bessel functions and modular relations of higher type and hyperbolic differential equations. Comm. Sém. Math. Univ. Lund [Medd. Lunds Univ. Mat. Sem.] 1952 (Tome Supplementaire), pp. 12–20.

ⓘ

External Links:

MathReview (S. C. van Veen), ZentralBlatt

Referenced by:

§35.5(i).

Encodings:

BibTeX

…

5: Bibliography M

… ►

Calculation of the modified Bessel functions of the second kind with complex argument. Math. Comp. 20 (95), pp. 407–412.

ⓘ

External Links:

ISSN 0025-5718, FullText, MathReview (H. E. Fettis), ZentralBlatt

Referenced by:

§10.74(iii).

Encodings:

BibTeX

… ►

Infinite families of exact sums of squares formulas, Jacobi elliptic functions, continued fractions, and Schur functions. Ramanujan J. 6 (1), pp. 7–149.

ⓘ

External Links:

Document, ZentralBlatt

Referenced by:

§27.13(iv).

Encodings:

BibTeX

… ►

New infinite families of exact sums of squares formulas, Jacobi elliptic functions, and Ramanujan’s tau function. Proc. Nat. Acad. Sci. U.S.A. 93 (26), pp. 15004–15008.

ⓘ

External Links:

ISSN 0027-8424, MathReview (Ken Ono), ZentralBlatt

Referenced by:

§27.13(iv).

Encodings:

BibTeX

… ►

The $q$-analogue of the Laguerre polynomials. J. Math. Anal. Appl. 81 (1), pp. 20–47.

ⓘ

External Links:

ISSN 0022-247X, Document, MathReview (R. A. Askey), ZentralBlatt

Referenced by:

§18.27(v).

Encodings:

BibTeX

… ►

Tata Lectures on Theta. II. Birkhäuser Boston Inc., Boston, MA.

ⓘ

Notes:

Jacobian theta functions and differential equations

External Links:

ISBN 0-8176-3110-0, MathReview (M. Kh. Gizatullin), ZentralBlatt

Referenced by:

§21.7(i), §21.7(ii), §21.7(iii), Ch.21.

Encodings:

BibTeX

…

6: Bibliography R

… ►

On the partition function p(n). Proc. London Math. Soc. (2) 43 (4), pp. 241–254.

ⓘ

External Links:

Document, MathReview Entry, ZentralBlatt

Referenced by:

§27.14(iii).

Encodings:

BibTeX

… ►

A non-negative representation of the linearization coefficients of the product of Jacobi polynomials. Canad. J. Math. 33 (4), pp. 915–928.

ⓘ

External Links:

ISSN 0008-414X, Document, Link, MathReview (R. A. Askey), ZentralBlatt

Referenced by:

§18.18(v).

Encodings:

BibTeX

… ►

Elliptic Functions, Theta Functions, and Riemann Surfaces. The Williams & Wilkins Co., Baltimore, MD.

ⓘ

External Links:

MathReview (H. H. Martens), ZentralBlatt

Referenced by:

§20.11(iv), §20.12(ii).

Encodings:

BibTeX

►

On the definition and properties of generalized $6$-$j$ symbols. J. Math. Phys. 20 (12), pp. 2398–2415.

ⓘ

External Links:

ISSN 0022-2488, Document, MathReview (S. Saran), ZentralBlatt

Referenced by:

§16.4(iii), §16.4(iii).

Encodings:

BibTeX

… ►

Infinite Sum of the Incomplete Gamma Function Expressed in Terms of the Hurwitz Zeta Function. Mathematics 9 (16).

ⓘ

External Links:

Link, ISSN 2227-7390, Document

Referenced by:

§8.15.

Encodings:

BibTeX

…

7: Bibliography I

… ►

Theta Functions. Springer-Verlag, New York.

ⓘ

Notes:

Die Grundlehren der mathematischen Wissenschaften, Band 194

External Links:

ISBN 3540056998, MathReview (H. Klingen), ZentralBlatt

Referenced by:

§21.5(ii), §21.8, Ch.21.

Encodings:

BibTeX

… ►

Tables of the elliptic cylinder functions. Proc. Roy. Soc. Edinburgh Sect. A 52, pp. 355–433.

ⓘ

External Links:

ZentralBlatt

Referenced by:

4th item, 2nd item.

Encodings:

BibTeX

… ►

The real roots of Bernoulli polynomials. Ann. Univ. Turku. Ser. A I 37, pp. 1–20.

ⓘ

External Links:

MathReview (D. H. Lehmer), ZentralBlatt

Referenced by:

§24.12(i).

Encodings:

BibTeX

… ►

Two families of orthogonal polynomials related to Jacobi polynomials. Rocky Mountain J. Math. 21 (1), pp. 359–375.

ⓘ

External Links:

ISSN 0035-7596, MathReview (Joaquin Bustoz), ZentralBlatt

Referenced by:

§18.30(i).

Encodings:

BibTeX

… ►

Asymptotics of the Askey-Wilson and $q$-Jacobi polynomials. SIAM J. Math. Anal. 17 (6), pp. 1475–1482.

ⓘ

External Links:

ISSN 0036-1410, Document, MathReview (David M. Bressoud), ZentralBlatt

Referenced by:

§18.29.

Encodings:

BibTeX

…

8: 18.5 Explicit Representations

… ►With $x=\cos \theta =\frac{1}{2}(z+z^{-1})$, … ►

§18.5(iii) Finite Power Series, the Hypergeometric Function, and Generalized Hypergeometric Functions

… ►

Jacobi

… ►The first of each of equations (18.5.7) and (18.5.8) can be regarded as definitions of $P_n^{(\alpha ,\beta )}\left(x\right)$ when the conditions $\alpha >-1$ and $\beta >-1$ are not satisfied. …For this reason, and also in the interest of simplicity, in the case of the Jacobi polynomials $P_n^{(\alpha ,\beta )}\left(x\right)$ we assume throughout this chapter that $\alpha >-1$ and $\beta >-1$, unless stated otherwise. …

9: Bibliography D

… ►

Computing Riemann theta functions. Math. Comp. 73 (247), pp. 1417–1442.

ⓘ

Notes:

Two of the authors (Deconinck and van Hoeij) have developed Maple code for the algorithms described in this paper.

External Links:

ISSN 0025-5718, Document, Code, MathReview, ZentralBlatt

Referenced by:

§21.10(i), 1st item, §21.2(i), §21.4, M. Heil (1995).

Encodings:

BibTeX

… ►

Complex zeros of cylinder functions. Math. Comp. 20 (94), pp. 215–222.

ⓘ

Notes:

For a numerical error in one of the zeros see Amos (1985).

External Links:

ISSN 0025-5718, FullText, MathReview, ZentralBlatt

Referenced by:

2nd item.

Encodings:

BibTeX

… ►

Uniform asymptotic expansions for Whittaker’s confluent hypergeometric functions. SIAM J. Math. Anal. 20 (3), pp. 744–760.

ⓘ

Notes:

(This reference has several typographical errors.)

External Links:

ISSN 0036-1410, Document, MathReview (F. W. J. Olver), ZentralBlatt

Referenced by:

§13.21(ii), §13.21(iii), §13.21(iv).

Encodings:

BibTeX

… ►

Asymptotic approximations for the Jacobi and ultraspherical polynomials, and related functions. Methods Appl. Anal. 6 (3), pp. 21–56.

ⓘ

External Links:

ISSN 1073-2772, Pdf, MathReview (Walter Van Assche), ZentralBlatt

Referenced by:

§15.12(iii), §18.15(i), §18.15(vi).

Encodings:

BibTeX

… ►

Product formulas and Nicholson-type integrals for Jacobi functions. I. Summary of results. SIAM J. Math. Anal. 9 (1), pp. 76–86.

ⓘ

External Links:

Document, MathReview (R. C. Varma), ZentralBlatt

Referenced by:

§18.17(iii).

Encodings:

BibTeX

…

10: Errata

… ►

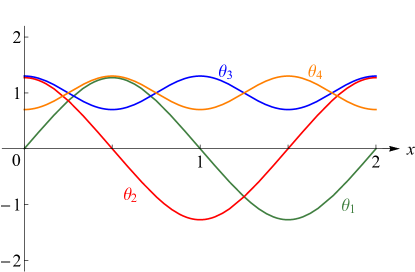
Figure 20.3.1 ${\theta }_j(\pi x,0.15)$, $0\le x\le 2$, $j=1,2,3,4$.

The locations of the tick marks denoting $1.5$ and $2$ on the $x$-axis were corrected.

Reported 2017-05-22 by Paul Abbott.

… ►

22.19.2 $$\sin \left(\frac{1}{2}\theta (t)\right)=\sin \left(\frac{1}{2}\alpha \right)\mathrm{sn}(t+K,\sin \left(\frac{1}{2}\alpha \right))$$

Originally the first argument to the function $\mathrm{sn}$ was given incorrectly as $t$. The correct argument is $t+K$.

Reported 2014-03-05 by Svante Janson.

►

22.19.3 $$\theta (t)=2\mathrm{am}(t\sqrt{E/2},\sqrt{2/E})$$

Originally the first argument to the function $\mathrm{am}$ was given incorrectly as $t$. The correct argument is $t\sqrt{E/2}$.

Reported 2014-03-05 by Svante Janson.

… ►

Originally a minus sign was missing in the entries for $\mathrm{cd}u$ and $\mathrm{dc}u$ in the second column (headed $z+K+i{K}^{\prime }$). The correct entries are $-k^{-1}\mathrm{ns}z$ and $-k\mathrm{sn}z$. Note: These entries appear online but not in the published print edition. More specifically, Table 22.4.3 in the published print edition is restricted to the three Jacobian elliptic functions $\mathrm{sn},\mathrm{cn},\mathrm{dn}$, whereas Table 22.4.3 covers all 12 Jacobian elliptic functions.

	$u$
	$z+K$	$z+K+\mathrm{i}{K}^{\prime }$	$z+\mathrm{i}{K}^{\prime }$	$z+2K$	$z+2K+2\mathrm{i}{K}^{\prime }$	$z+2\mathrm{i}{K}^{\prime }$
$\mathrm{⋮}$
$\mathrm{cd}u$	$-\mathrm{sn}z$	$-k^{-1}\mathrm{ns}z$	$k^{-1}\mathrm{dc}z$	$-\mathrm{cd}z$	$-\mathrm{cd}z$	$\mathrm{cd}z$
$\mathrm{⋮}$
$\mathrm{dc}u$	$-\mathrm{ns}z$	$-k\mathrm{sn}z$	$k\mathrm{cd}z$	$-\mathrm{dc}z$	$-\mathrm{dc}z$	$\mathrm{dc}z$
$\mathrm{⋮}$

Reported 2014-02-28 by Svante Janson.

… ►

Several new equations have been added. See (8.17.24), (20.7.34), §20.11(v), (26.12.27), (36.2.28), and (36.2.29).

…