§21.7 Riemann Surfaces

Keywords:: Riemann surface
Permalink:: http://dlmf.nist.gov/21.7

§21.7(i) Connection of Riemann Theta Functions to Riemann Surfaces
§21.7(ii) Fay’s Trisecant Identity
§21.7(iii) Frobenius’ Identity

§21.7(i) Connection of Riemann Theta Functions to Riemann Surfaces

Keywords:: Riemann surface, algebraic curves, cycle, genus, handle, plane algebraic curves
Referenced by:: §21.1, Figure 21.4.5, Figure 21.4.5, §21.4
Permalink:: http://dlmf.nist.gov/21.7.i

In almost all applications, a Riemann theta function is associated with a compact Riemann surface. Although there are other ways to represent Riemann surfaces (see e.g. Belokolos et al. (1994, §2.1)), they are obtainable from plane algebraic curves (Springer (1957), or Riemann (1851)). Consider the set of points in $\Complex^{2}$ that satisfy the equation

21.7.1 $P(\lambda,\mu)=0,$

Symbols:: $P(\lambda,\mu)$ : polynomial
Referenced by:: §21.7(i)
Permalink:: http://dlmf.nist.gov/21.7.E1
Encodings:: TeX, pMML, png

where $P(\lambda,\mu)$ is a polynomial in $\lambda$ and $\mu$ that does not factor over $\Complex^{2}$ . Equation (21.7.1) determines a plane algebraic curve in $\Complex^{2}$ , which is made compact by adding its points at infinity. To accomplish this we write (21.7.1) in terms of homogeneous coordinates:

21.7.2 $\tilde{P}(\tilde{\lambda},\tilde{\mu},\tilde{\eta})=0,$

Symbols:: $P(\lambda,\mu)$ : polynomial
Permalink:: http://dlmf.nist.gov/21.7.E2
Encodings:: TeX, pMML, png

by setting $\lambda=\tilde{\lambda}/\tilde{\eta}$ , $\mu=\tilde{\mu}/\tilde{\eta}$ , and then clearing fractions. This compact curve may have singular points, that is, points at which the gradient of $\tilde{P}$ vanishes. Removing the singularities of this curve gives rise to a two-dimensional connected manifold with a complex-analytic structure, that is, a Riemann surface. All compact Riemann surfaces can be obtained this way.

Since a Riemann surface $\Gamma$ is a two-dimensional manifold that is orientable (owing to its analytic structure), its only topological invariant is its genus $g$ (the number of handles in the surface). On this surface, we choose $2g$ cycles (that is, closed oriented curves, each with at most a finite number of singular points) $a_{j}$ , $b_{j}$ , $j=1,2,\dots,g$ , such that their intersection indices satisfy

21.7.3

$a_{j}\circ a_{k}=0,$

$b_{j}\circ b_{k}=0,$

$a_{j}\circ b_{k}=\delta _{{j,k}}.$

Symbols:: $\delta _{{j,k}}$ : Kronecker delta, $a_{j}$ : cycles and $b_{j}$ : cycles
Permalink:: http://dlmf.nist.gov/21.7.E3
Encodings:: TeX, TeX, TeX, pMML, pMML, pMML, png, png, png

For example, Figure 21.7.1 depicts a genus 2 surface.

Figure 21.7.1: A basis of cycles for a genus 2 surface.

Referenced by:: §21.7(i)
Permalink:: http://dlmf.nist.gov/21.7.F1
Encodings:: pdf, png

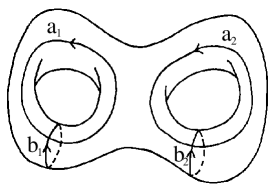
On a Riemann surface of genus $g$ , there are $g$ linearly independent holomorphic differentials $\omega _{j}$ , $j=1,2,\dots,g$ . If a local coordinate $z$ is chosen on the Riemann surface, then the local coordinate representation of these holomorphic differentials is given by

21.7.4 $\omega _{j}=f_{j}(z)dz,$ $j=1,2,\dots,g$ ,

Symbols:: $dx$ : differential of $x$ , $g$ : positive integer, $\omega$ : differential and $f_{j}(z)$ : analytic functions
Permalink:: http://dlmf.nist.gov/21.7.E4
Encodings:: TeX, pMML, png

where $f_{j}(z)$ , $j=1,2,\dots,g$ are analytic functions. Thus the differentials $\omega _{j}$ , $j=1,2,\dots,g$ have no singularities on $\Gamma$ . Note that for the purposes of integrating these holomorphic differentials, all cycles on the surface are a linear combination of the cycles $a_{j}$ , $b_{j}$ , $j=1,2,\dots,g$ . The $\omega _{j}$ are normalized so that

21.7.5 $\oint _{{a_{k}}}\omega _{j}=\delta _{{j,k}},$ $j,k=1,2,\dots,g$ .

Symbols:: $\delta _{{j,k}}$ : Kronecker delta, $g$ : positive integer, $\omega$ : differential and $a_{j}$ : cycles
Permalink:: http://dlmf.nist.gov/21.7.E5
Encodings:: TeX, pMML, png

Then the matrix defined by

21.7.6 $\Omega _{{jk}}=\oint _{{b_{k}}}\omega _{j},$ $j,k=1,2,\dots,g$ ,

Symbols:: $g$ : positive integer, $\omega$ : differential and $b_{j}$ : cycles
Permalink:: http://dlmf.nist.gov/21.7.E6
Encodings:: TeX, pMML, png

is a Riemann matrix and it is used to define the corresponding Riemann theta function. In this way, we associate a Riemann theta function with every compact Riemann surface $\Gamma$ .

Riemann theta functions originating from Riemann surfaces are special in the sense that a general $g$ -dimensional Riemann theta function depends on $g(g+1)/2$ complex parameters. In contrast, a $g$ -dimensional Riemann theta function arising from a compact Riemann surface of genus $g$ ( $>1$ ) depends on at most $3g-3$ complex parameters (one complex parameter for the case $g=1$ ). These special Riemann theta functions satisfy many special identities, two of which appear in the following subsections. For more information, see Dubrovin (1981), Brieskorn and Knörrer (1986, §9.3), Belokolos et al. (1994, Chapter 2), and Mumford (1984, §2.2–2.3).

§21.7(ii) Fay’s Trisecant Identity

Notes:: See Mumford (1984, pp. 207-260).
Keywords:: Fay’s trisecant identity, Riemann surface, Riemann theta functions with characteristics
Permalink:: http://dlmf.nist.gov/21.7.ii

Let $\boldsymbol{{\alpha}}$ , $\boldsymbol{{\beta}}$ be such that

21.7.7 $\left(\frac{\partial}{\partial z_{1}}\mathop{\theta\!\genfrac{[}{]}{0.0pt}{}{\boldsymbol{{\alpha}}}{\boldsymbol{{\beta}}}\/}\nolimits\!\left(\mathbf{z}\middle|\boldsymbol{{\Omega}}\right)\Big|_{{\mathbf{z}=\boldsymbol{{0}}}},\dots,\frac{\partial}{\partial z_{g}}\mathop{\theta\!\genfrac{[}{]}{0.0pt}{}{\boldsymbol{{\alpha}}}{\boldsymbol{{\beta}}}\/}\nolimits\!\left(\mathbf{z}\middle|\boldsymbol{{\Omega}}\right)\Big|_{{\mathbf{z}=\boldsymbol{{0}}}}\right)\neq\boldsymbol{{0}}.$

Symbols:: $\mathop{\theta\!\genfrac{[}{]}{0.0pt}{}{\boldsymbol{{\alpha}}}{\boldsymbol{{\beta}}}\/}\nolimits\!\left(\mathbf{z}\middle|\boldsymbol{{\Omega}}\right)$ : Riemann theta function with characteristics, $\frac{\partial f}{\partial x}$ : partial derivative of $f$ with respect to $x$ , $g$ : positive integer, $\boldsymbol{{\Omega}}$ : a Riemann matrix, $\boldsymbol{{\alpha}}$ : $g$ -dimensional vector and $\boldsymbol{{\beta}}$ : $g$ -dimensional vector
Permalink:: http://dlmf.nist.gov/21.7.E7
Encodings:: TeX, pMML, png

Define the holomorphic differential

21.7.8 $\zeta=\sum _{{j=1}}^{g}\omega _{j}\frac{\partial}{\partial z_{j}}\mathop{\theta\!\genfrac{[}{]}{0.0pt}{}{\boldsymbol{{\alpha}}}{\boldsymbol{{\beta}}}\/}\nolimits\!\left(\mathbf{z}\middle|\boldsymbol{{\Omega}}\right)\Big|_{{\mathbf{z}=\boldsymbol{{0}}}}.$

Symbols:: $\mathop{\theta\!\genfrac{[}{]}{0.0pt}{}{\boldsymbol{{\alpha}}}{\boldsymbol{{\beta}}}\/}\nolimits\!\left(\mathbf{z}\middle|\boldsymbol{{\Omega}}\right)$ : Riemann theta function with characteristics, $\frac{\partial f}{\partial x}$ : partial derivative of $f$ with respect to $x$ , $g$ : positive integer, $\boldsymbol{{\Omega}}$ : a Riemann matrix, $\boldsymbol{{\alpha}}$ : $g$ -dimensional vector, $\boldsymbol{{\beta}}$ : $g$ -dimensional vector and $\omega$ : differential
Permalink:: http://dlmf.nist.gov/21.7.E8
Encodings:: TeX, pMML, png

Then the prime form on the corresponding compact Riemann surface $\Gamma$ is defined by

21.7.9 $E(P_{1},P_{2})=\mathop{\theta\!\genfrac{[}{]}{0.0pt}{}{\boldsymbol{{\alpha}}}{\boldsymbol{{\beta}}}\/}\nolimits\!\left(\int _{{P_{1}}}^{{P_{2}}}\boldsymbol{{\omega}}\middle|\boldsymbol{{\Omega}}\right)\Bigg/\left(\sqrt{\zeta(P_{1})}\sqrt{\zeta(P_{2})}\right),$

Symbols:: $\mathop{\theta\!\genfrac{[}{]}{0.0pt}{}{\boldsymbol{{\alpha}}}{\boldsymbol{{\beta}}}\/}\nolimits\!\left(\mathbf{z}\middle|\boldsymbol{{\Omega}}\right)$ : Riemann theta function with characteristics, $\int$ : integral, $(a,b)$ : open interval, $\boldsymbol{{\Omega}}$ : a Riemann matrix, $\boldsymbol{{\alpha}}$ : $g$ -dimensional vector, $\boldsymbol{{\beta}}$ : $g$ -dimensional vector, $E(P_{1},P_{2})$ : prime form, $P_{1},P_{2}$ : points and $\boldsymbol{{\omega}}$ : vector of differentials
Permalink:: http://dlmf.nist.gov/21.7.E9
Encodings:: TeX, pMML, png

where $P_{1}$ and $P_{2}$ are points on $\Gamma$ , $\boldsymbol{{\omega}}=(\omega _{1},\omega _{2},\dots,\omega _{g})$ , and the path of integration on $\Gamma$ from $P_{1}$ to $P_{2}$ is identical for all components. Here $\sqrt{\zeta(P)}$ is such that $\sqrt{\zeta(P)}^{2}=\zeta(P)$ , $P\in\Gamma$ . Either branch of the square roots may be chosen, as long as the branch is consistent across $\Gamma$ . For all $\mathbf{z}\in\Complex^{g}$ , and all $P_{1}$ , $P_{2}$ , $P_{3}$ , $P_{4}$ on $\Gamma$ , Fay’s identity is given by

21.7.10 $\mathop{\theta\/}\nolimits\!\left(\mathbf{z}+\int _{{P_{1}}}^{{P_{3}}}\boldsymbol{{\omega}}\middle|\boldsymbol{{\Omega}}\right)\mathop{\theta\/}\nolimits\!\left(\mathbf{z}+\int _{{P_{2}}}^{{P_{4}}}\boldsymbol{{\omega}}\middle|\boldsymbol{{\Omega}}\right)E(P_{3},P_{2})E(P_{1},P_{4})+\mathop{\theta\/}\nolimits\!\left(\mathbf{z}+\int _{{P_{2}}}^{{P_{3}}}\boldsymbol{{\omega}}\middle|\boldsymbol{{\Omega}}\right)\mathop{\theta\/}\nolimits\!\left(\mathbf{z}+\int _{{P_{1}}}^{{P_{4}}}\boldsymbol{{\omega}}\middle|\boldsymbol{{\Omega}}\right)E(P_{3},P_{1})E(P_{4},P_{2})=\mathop{\theta\/}\nolimits\!\left(\mathbf{z}\middle|\boldsymbol{{\Omega}}\right)\mathop{\theta\/}\nolimits\!\left(\mathbf{z}+\int _{{P_{1}}}^{{P_{3}}}\boldsymbol{{\omega}}+\int _{{P_{2}}}^{{P_{4}}}\boldsymbol{{\omega}}\middle|\boldsymbol{{\Omega}}\right)E(P_{1},P_{2})E(P_{3},P_{4}),$

Symbols:: $\mathop{\theta\/}\nolimits\!\left(\mathbf{z}\middle|\boldsymbol{{\Omega}}\right)$ : Riemann theta function, $\int$ : integral, $(a,b)$ : open interval, $\boldsymbol{{\Omega}}$ : a Riemann matrix, $E(P_{1},P_{2})$ : prime form, $P_{1},P_{2}$ : points and $\boldsymbol{{\omega}}$ : vector of differentials
Referenced by:: §21.7(ii)
Permalink:: http://dlmf.nist.gov/21.7.E10
Encodings:: TeX, pMML, png

where again all integration paths are identical for all components. Generalizations of this identity are given in Fay (1973, Chapter 2). Fay derives (21.7.10) as a special case of a more general class of addition theorems for Riemann theta functions on Riemann surfaces.

§21.7(iii) Frobenius’ Identity

Notes:: See Mumford (1984, pp. 106–120).
Keywords:: Frobenius’ identity, Riemann surface, Riemann theta functions with characteristics, algebraic curves
Referenced by:: §20.11(iv)
Permalink:: http://dlmf.nist.gov/21.7.iii

Let $\Gamma$ be a hyperelliptic Riemann surface. These are Riemann surfaces that may be obtained from algebraic curves of the form

21.7.11 $\mu^{2}=Q(\lambda),$

Symbols:: $Q(\lambda)$ : polynomial
Permalink:: http://dlmf.nist.gov/21.7.E11
Encodings:: TeX, pMML, png

where $Q(\lambda)$ is a polynomial in $\lambda$ of odd degree $2g+1$ $(\geq 5)$ . The genus of this surface is $g$ . The zeros $\lambda _{j}$ , $j=1,2,\dots,2g+1$ of $Q(\lambda)$ specify the finite branch points $P_{j}$ , that is, points at which $\mu _{j}=0$ , on the Riemann surface. Denote the set of all branch points by $B=\{ P_{1},P_{2},\dots,P_{{2g+1}},P_{\infty}\}$ . Consider a fixed subset $U$ of $B$ , such that the number of elements $|U|$ in the set $U$ is $g+1$ , and $P_{\infty}\notin U$ . Next, define an isomorphism $\boldsymbol{{\eta}}$ which maps every subset $T$ of $B$ with an even number of elements to a $2g$ -dimensional vector $\boldsymbol{{\eta}}(T)$ with elements either 0 or $\tfrac{1}{2}$ . Define the operation

21.7.12 $T_{1}\ominus T_{2}=(T_{1}\cup T_{2})\setminus(T_{1}\cap T_{2}).$

Symbols:: $\cap$ : intersection, $\setminus$ : set subtraction, $\cup$ : union and $T$ : subset of $B$
Permalink:: http://dlmf.nist.gov/21.7.E12
Encodings:: TeX, pMML, png

Also, $T^{c}=B\setminus T$ , $\boldsymbol{{\eta}}^{1}(T)=(\eta _{1}(T),\eta _{2}(T),\dots,\eta _{g}(T))$ , and $\boldsymbol{{\eta}}^{2}(T)=(\eta _{{g+1}}(T),\eta _{{g+2}}(T),\dots,\eta _{{2g}}(T))$ . Then the isomorphism is determined completely by:

21.7.13 $\boldsymbol{{\eta}}(T)=\boldsymbol{{\eta}}(T^{c}),$

Symbols:: $T$ : subset of $B$
Permalink:: http://dlmf.nist.gov/21.7.E13
Encodings:: TeX, pMML, png

21.7.14 $\boldsymbol{{\eta}}(T_{1}\ominus T_{2})=\boldsymbol{{\eta}}(T_{1})+\boldsymbol{{\eta}}(T_{2}),$

Symbols:: $T$ : subset of $B$
Permalink:: http://dlmf.nist.gov/21.7.E14
Encodings:: TeX, pMML, png

21.7.15 $4\boldsymbol{{\eta}}^{1}(T)\cdot\boldsymbol{{\eta}}^{2}(T)=\tfrac{1}{2}\left(|T\ominus U|-g-1\right)\;\;(\mathop{{\rm mod}}2),$

Symbols:: $g$ : positive integer, $U$ : subset of $B$ and $T$ : subset of $B$
Permalink:: http://dlmf.nist.gov/21.7.E15
Encodings:: TeX, pMML, png

21.7.16 $4(\boldsymbol{{\eta}}^{1}(T_{1})\cdot\boldsymbol{{\eta}}^{2}(T_{2})-\boldsymbol{{\eta}}^{2}(T_{1})\cdot\boldsymbol{{\eta}}^{1}(T_{2}))=|T_{1}\cap T_{2}|\;\;(\mathop{{\rm mod}}2).$

Symbols:: $\cap$ : intersection and $T$ : subset of $B$
Permalink:: http://dlmf.nist.gov/21.7.E16
Encodings:: TeX, pMML, png

Furthermore, let $\boldsymbol{{\eta}}(P_{\infty})=\boldsymbol{{0}}$ and $\boldsymbol{{\eta}}(P_{j})=\boldsymbol{{\eta}}(\{ P_{j},P_{\infty}\})$ . Then for all $\mathbf{z}_{j}\in\Complex^{g}$ , $j=1,2,3,4$ , such that $\mathbf{z}_{1}+\mathbf{z}_{2}+\mathbf{z}_{3}+\mathbf{z}_{4}=0$ , and for all $\boldsymbol{{\alpha}}_{j}$ , $\boldsymbol{{\beta}}_{j}$ $\in\Real^{g}$ , such that $\boldsymbol{{\alpha}}_{1}+\boldsymbol{{\alpha}}_{2}+\boldsymbol{{\alpha}}_{3}+\boldsymbol{{\alpha}}_{4}=0$ and $\boldsymbol{{\beta}}_{1}+\boldsymbol{{\beta}}_{2}+\boldsymbol{{\beta}}_{3}+\boldsymbol{{\beta}}_{4}=0$ , we have Frobenius’ identity:

21.7.17 $\sum _{{P_{j}\in U}}\prod _{{k=1}}^{4}\mathop{\theta\!\genfrac{[}{]}{0.0pt}{}{\boldsymbol{{\alpha}}_{k}+\boldsymbol{{\eta}}^{1}(P_{j})}{\boldsymbol{{\beta}}_{k}+\boldsymbol{{\eta}}^{2}(P_{j})}\/}\nolimits\!\left(\mathbf{z}_{k}\middle|\boldsymbol{{\Omega}}\right)=\sum _{{P_{j}\in U^{c}}}\prod _{{k=1}}^{4}\mathop{\theta\!\genfrac{[}{]}{0.0pt}{}{\boldsymbol{{\alpha}}_{k}+\boldsymbol{{\eta}}^{1}(P_{j})}{\boldsymbol{{\beta}}_{k}+\boldsymbol{{\eta}}^{2}(P_{j})}\/}\nolimits\!\left(\mathbf{z}_{k}\middle|\boldsymbol{{\Omega}}\right).$

Symbols:: $\mathop{\theta\!\genfrac{[}{]}{0.0pt}{}{\boldsymbol{{\alpha}}}{\boldsymbol{{\beta}}}\/}\nolimits\!\left(\mathbf{z}\middle|\boldsymbol{{\Omega}}\right)$ : Riemann theta function with characteristics, $\in$ : element of, $\boldsymbol{{\Omega}}$ : a Riemann matrix, $\boldsymbol{{\alpha}}$ : $g$ -dimensional vector, $\boldsymbol{{\beta}}$ : $g$ -dimensional vector, $P_{j}$ : branch points and $U$ : subset of $B$
Permalink:: http://dlmf.nist.gov/21.7.E17
Encodings:: TeX, pMML, png

§21.7 Riemann Surfaces

Contents

§21.7(i) Connection of Riemann Theta Functions to Riemann Surfaces

§21.7(ii) Fay’s Trisecant Identity

§21.7(iii) Frobenius’ Identity