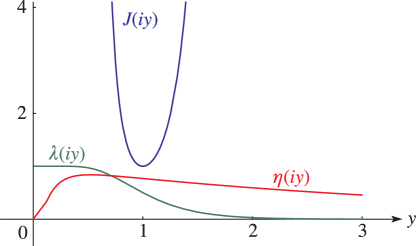
real and imaginary parts

SearchAdvancedHelp

(0.007 seconds)

21—30 of 180 matching pages

21: 5.7 Series Expansions

… ►

5.7.8 $$\mathrm{\Im }\psi \left(1+\mathrm{i}y\right)=\sum _{k=1}^{\mathrm{\infty }}\frac{y}{k^2+y^2}.$$

ⓘ

Symbols:

$\psi \left(z\right)$: psi (or digamma) function, $\mathrm{\Im }$: imaginary part, $\mathrm{i}$: imaginary unit, $k$: nonnegative integer and $y$: real variable

A&S Ref:

6.3.13

Permalink:

http://dlmf.nist.gov/5.7.E8

Encodings:

pMML, png, TeX

See also:

Annotations for §5.7(ii), §5.7 and Ch.5

22: 23.22 Methods of Computation

… ►This yields a pair of generators that satisfy $\mathrm{\Im }\tau >0$, $|\mathrm{\Re }\tau |\le \frac{1}{2}$, $|\tau |>1$. … ►

In the general case, given by $cd\ne 0$, we compute the roots $\alpha$, $\beta$, $\gamma$, say, of the cubic equation $4t^3-ct-d=0$; see §1.11(iii). These roots are necessarily distinct and represent $e_1$, $e_2$, $e_3$ in some order.

If $c$ and $d$ are real, and the discriminant is positive, that is $c^3-27d^2>0$, then $e_1$, $e_2$, $e_3$ can be identified via (23.5.1), and $k^2$, $k_{}^{\prime }{}_{}{}^2$ obtained from (23.6.16).

If , or $c$ and $d$ are not both real, then we label $\alpha$, $\beta$, $\gamma$ so that the triangle with vertices $\alpha$, $\beta$, $\gamma$ is positively oriented and $[\alpha ,\gamma ]$ is its longest side (chosen arbitrarily if there is more than one). In particular, if $\alpha$, $\beta$, $\gamma$ are collinear, then we label them so that $\beta$ is on the line segment $(\alpha ,\gamma )$. In consequence, $k^2=(\beta -\gamma )/(\alpha -\gamma )$, $k_{}^{\prime }{}_{}{}^2=(\alpha -\beta )/(\alpha -\gamma )$ satisfy $\mathrm{\Im }{k}^2\ge 0\ge \mathrm{\Im }k_{}^{\prime }{}_{}{}^2$ (with strict inequality unless $\alpha$, $\beta$, $\gamma$ are collinear); also $|k^2|$, $|k_{}^{\prime }{}_{}{}^2|\le 1$.

Finally, on taking the principal square roots of $k^2$ and $k_{}^{\prime }{}_{}{}^2$ we obtain values for $k$ and $k^{\prime }$ that lie in the 1st and 4th quadrants, respectively, and $2{\omega }_1$, $2{\omega }_3$ are given by

23.22.1 $$2{\omega }_{1}M(1,k^{\prime })=-2\mathrm{i}{\omega }_{3}M(1,k)=\frac{\pi }{3}\sqrt{\frac{c(2+k^{2}k_{}^{\prime }{}_{}{}^2)(k_{}^{\prime }{}_{}{}^2-k^2)}{d(1-k^{2}k_{}^{\prime }{}_{}{}^2)}},$$

ⓘ

Symbols:

$M(a,g)$: arithmetic-geometric mean, $\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{i}$: imaginary unit, ${\omega }_1$, ${\omega }_3$, ${\omega }_2=-{\omega }_1-{\omega }_3$: lattice generators, $k$: modulus and $k^{\prime }$: complementary modulus

Proof sketch:

Combine (23.6.16), (23.6.17), (22.20.6), (23.3.5)–(23.3.7), and use analytical continuation.

Referenced by:

§23.22(ii)

Permalink:

http://dlmf.nist.gov/23.22.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §23.22(ii), §23.22(ii), §23.22 and Ch.23

where $M$ denotes the arithmetic-geometric mean (see §§19.8(i) and 22.20(ii)). This process yields 2 possible pairs ($2{\omega }_1$, $2{\omega }_3$), corresponding to the 2 possible choices of the square root.

…

23: 21.2 Definitions

… ►

21.2.2 $$\widehat{\theta }\left(𝐳\right|𝛀)={\mathrm{e}}^{-\pi [\mathrm{\Im }𝐳]\cdot {[\mathrm{\Im }𝛀]}^{-1}\cdot [\mathrm{\Im }𝐳]}\theta \left(𝐳\right|𝛀).$$

ⓘ

Defines:

$\widehat{\theta }\left(𝐳\right|𝛀)$: scaled Riemann theta function

Symbols:

$\theta \left(𝐳\right|𝛀)$: Riemann theta function, $\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{e}$: base of natural logarithm, $\mathrm{\Im }$: imaginary part and $𝛀$: a Riemann matrix

Permalink:

http://dlmf.nist.gov/21.2.E2

Encodings:

pMML, png, TeX

See also:

Annotations for §21.2(i), §21.2 and Ch.21

… ►

21.2.3

ⓘ

Symbols:

$\theta \left(𝐳\right|𝛀)$: Riemann theta function, $\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{e}$: base of natural logarithm and $\mathrm{i}$: imaginary unit

Permalink:

http://dlmf.nist.gov/21.2.E3

Encodings:

pMML, png, TeX

See also:

Annotations for §21.2(i), §21.2(i), §21.2 and Ch.21

►With $z_1=x_1+\mathrm{i}{y}_1$, $z_2=x_2+\mathrm{i}{y}_2$, ►

21.2.4

ⓘ

Symbols:

$\widehat{\theta }\left(𝐳\right|𝛀)$: scaled Riemann theta function, $\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{e}$: base of natural logarithm and $\mathrm{i}$: imaginary unit

Permalink:

http://dlmf.nist.gov/21.2.E4

Encodings:

pMML, png, TeX

See also:

Annotations for §21.2(i), §21.2(i), §21.2 and Ch.21

… ►Let $𝜶,𝜷\in {ℝ}^g$. …

24: 4.3 Graphics

… ►Figure 4.3.2 illustrates the conformal mapping of the strip onto the whole $w$-plane cut along the negative real axis, where $w={\mathrm{e}}^z$ and $z=\ln w$ (principal value). …

25: 23.5 Special Lattices

… ►This occurs when ${\omega }_1$ is real and positive, $\mathrm{\Im }{\omega }_3>0$, $\mathrm{\Re }{\omega }_3=\frac{1}{2}{\omega }_1$, and . … ►The lattice root $e_1$ is real, and $e_3=\overline{e_2}$, with $\mathrm{\Im }{e}_2>0$. …

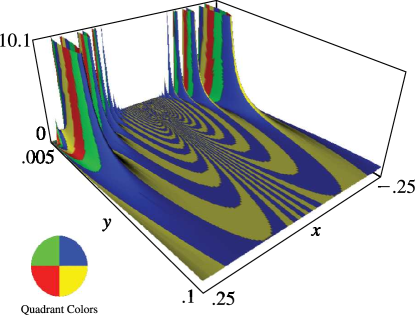
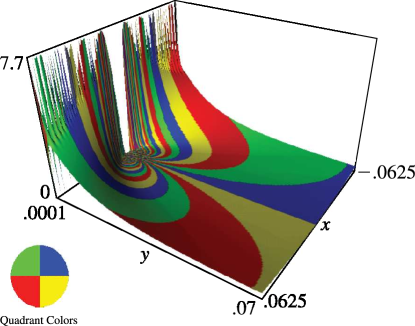
26: 23.16 Graphics

… ►

►►

Figure 23.16.1: Modular functions $\lambda \left(\mathrm{i}y\right)$, $J\left(\mathrm{i}y\right)$, $\eta \left(\mathrm{i}y\right)$ for $0\le y\le 3$. … Magnify

►

►

►

Figure 23.16.2: Elliptic modular function $\lambda \left(x+\mathrm{i}y\right)$ for $-0.25\le x\le 0.25$, $0.005\le y\le 0.1$. Magnify 3D Help

►

►

►

Figure 23.16.3: Dedekind’s eta function $\eta \left(x+\mathrm{i}y\right)$ for $-0.0625\le x\le 0.0625$, $0.0001\le y\le 0.07$. Magnify 3D Help

27: 9.18 Tables

… ►

Woodward and Woodward (1946) tabulates the real and imaginary parts of $\mathrm{Ai}\left(z\right)$, ${\mathrm{Ai}}^{\prime }\left(z\right)$, $\mathrm{Bi}\left(z\right)$, ${\mathrm{Bi}}^{\prime }\left(z\right)$ for $\mathrm{\Re }z=-2.4(.2)2.4$, $\mathrm{\Im }z=-2.4(.2)0$. Precision is 4D.

►

Harvard University (1945) tabulates the real and imaginary parts of $h_1(z)$, $h_1^{\prime }(z)$, $h_2(z)$, $h_2^{\prime }(z)$ for $-x_0\le \mathrm{\Re }z\le x_0$, $0\le \mathrm{\Im }z\le y_0$, , with interval 0.1 in $\mathrm{\Re }z$ and $\mathrm{\Im }z$. Precision is 8D. Here $h_1(z)=-2^{4/3}{3}^{1/6}\mathrm{i}\mathrm{Ai}\left({\mathrm{e}}^{-\pi \mathrm{i}/3}z\right)$, $h_2(z)=2^{4/3}{3}^{1/6}\mathrm{i}\mathrm{Ai}\left({\mathrm{e}}^{\pi \mathrm{i}/3}z\right)$.

… ►

Corless et al. (1992) gives the real and imaginary parts of ${\beta }_k$ for $k=1(1)13$; 14S.

… ►

Nosova and Tumarkin (1965) tabulates $e_0(x)\equiv \pi \mathrm{Hi}\left(-x\right)$, $e_0^{\prime }(x)=-\pi {\mathrm{Hi}}^{\prime }\left(-x\right)$, ${\tilde{e}}_0(-x)\equiv -\pi \mathrm{Gi}\left(x\right)$, ${\tilde{e}}_0^{\prime }(-x)=\pi {\mathrm{Gi}}^{\prime }\left(x\right)$ for $x=-1(.01)10$; 7D. Also included are the real and imaginary parts of $e_0(z)$ and $\mathrm{i}{e}_0^{\prime }(z)$, where $z=\mathrm{i}y$ and $y=0(.01)9$; 6-7D.

…

28: 4.37 Inverse Hyperbolic Functions

… ►In (4.37.1) the integration path may not pass through either of the points $t=\pm \mathrm{i}$, and the function ${(1+t^2)}^{1/2}$ assumes its principal value when $t$ is real. … ►Each is two-valued on the corresponding cut(s), and each is real on the part of the real axis that remains after deleting the intersections with the corresponding cuts. … ►the upper or lower sign being taken according as $\mathrm{\Re }z\gtrless 0$; compare Figure 4.37.1(ii). … ►On the part of the cuts from $-1$ to $1$ … ►On the part of the cut from $-\mathrm{\infty }$ to $-1$ …

29: 10.43 Integrals

… ►

10.43.26 $${\int }_0^{\mathrm{\infty }}\frac{K_{\mu }\left(at\right)J_{\nu }\left(bt\right)}{t^{\lambda }}dt=\frac{b^{\nu }\mathrm{\Gamma }\left(\frac{1}{2}\nu -\frac{1}{2}\lambda +\frac{1}{2}\mu +\frac{1}{2}\right)\mathrm{\Gamma }\left(\frac{1}{2}\nu -\frac{1}{2}\lambda -\frac{1}{2}\mu +\frac{1}{2}\right)}{2^{\lambda +1}{a}^{\nu -\lambda +1}}𝐅(\frac{\nu -\lambda +\mu +1}{2},\frac{\nu -\lambda -\mu +1}{2};\nu +1;-\frac{b^2}{a^2}),$$ $\mathrm{\Re }\left(\nu +1-\lambda \right)>|\mathrm{\Re }\mu |,\mathrm{\Re }a>|\mathrm{\Im }b|$.

ⓘ

Symbols:

$J_{\nu }\left(z\right)$: Bessel function of the first kind, $\mathrm{\Gamma }\left(z\right)$: gamma function, $dx$: differential of $x$ , $\mathrm{\Im }$: imaginary part, $\int$: integral, $K_{\nu }\left(z\right)$: modified Bessel function of the second kind, $\mathrm{\Re }$: real part, $𝐅(a,b;c;z)$ or $𝐅(\genfrac{}{}{0pt}{}{a,b}{c};z)$: $={}_2{}^{}𝐅_1^{}(a,b;c;z)$ Olver’s hypergeometric function and $\nu$: complex parameter

Keywords:

Mellin transform

Permalink:

http://dlmf.nist.gov/10.43.E26

Encodings:

pMML, png, TeX

See also:

Annotations for §10.43(iv), §10.43 and Ch.10

… ►

10.43.27 $${\int }_0^{\mathrm{\infty }}{t}^{\mu +\nu +1}{K}_{\mu }\left(at\right)J_{\nu }\left(bt\right)dt=\frac{{(2a)}^{\mu }{(2b)}^{\nu }\mathrm{\Gamma }\left(\mu +\nu +1\right)}{{(a^2+b^2)}^{\mu +\nu +1}},$$ $\mathrm{\Re }\left(\nu +1\right)>|\mathrm{\Re }\mu |,\mathrm{\Re }a>|\mathrm{\Im }b|$.

ⓘ

Symbols:

$J_{\nu }\left(z\right)$: Bessel function of the first kind, $\mathrm{\Gamma }\left(z\right)$: gamma function, $dx$: differential of $x$ , $\mathrm{\Im }$: imaginary part, $\int$: integral, $K_{\nu }\left(z\right)$: modified Bessel function of the second kind, $\mathrm{\Re }$: real part and $\nu$: complex parameter

Keywords:

Mellin transform

Permalink:

http://dlmf.nist.gov/10.43.E27

Encodings:

pMML, png, TeX

See also:

Annotations for §10.43(iv), §10.43 and Ch.10

…

30: 10.7 Limiting Forms

… ►

10.7.7 $$H_{\nu }^{(1)}\left(z\right)\sim -H_{\nu }^{(2)}\left(z\right)\sim -(\mathrm{i}/\pi )\mathrm{\Gamma }\left(\nu \right){(\frac{1}{2}z)}^{-\nu },$$ $\mathrm{\Re }\nu >0$.

ⓘ

Symbols:

$\mathrm{\Gamma }\left(z\right)$: gamma function, $H_{\nu }^{(1)}\left(z\right)$: Bessel function of the third kind (or Hankel function), $H_{\nu }^{(2)}\left(z\right)$: Bessel function of the third kind (or Hankel function), $\sim$: asymptotic equality, $\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{i}$: imaginary unit, $\mathrm{\Re }$: real part, $z$: complex variable and $\nu$: complex parameter

Referenced by:

§10.7(i), §10.7(i)

Permalink:

http://dlmf.nist.gov/10.7.E7

Encodings:

pMML, png, TeX

See also:

Annotations for §10.7(i), §10.7 and Ch.10

…