painleve%C3%A9%20transcendents

SearchAdvancedHelp

(0.002 seconds)

11—20 of 138 matching pages

11: 32.5 Integral Equations

§32.5 Integral Equations

…

12: 32.4 Isomonodromy Problems

… ►Suppose … ►

§32.4(ii) First Painlevé Equation

… ►

§32.4(iii) Second Painlevé Equation

… ►

§32.4(iv) Third Painlevé Equation

… ►

13: Peter A. Clarkson

… ►Clarkson has published numerous papers on integrable systems (primarily Painlevé equations), special functions, and symmetry methods for differential equations. … ►

32 Painlevé Transcendents

…

14: 15.17 Mathematical Applications

… ►This topic is treated in §§15.10 and 15.11. ►The logarithmic derivatives of some hypergeometric functions for which quadratic transformations exist (§15.8(iii)) are solutions of Painlevé equations. … …

15: Bibliography I

… ►

On the asymptotic analysis of the Painlevé equations via the isomonodromy method. Nonlinearity 7 (5), pp. 1291–1325.

ⓘ

External Links:

ISSN 0951-7715, Document, MathReview (Alexander Vladimirovich Kitaev), ZentralBlatt

Referenced by:

§32.11(iii).

Encodings:

BibTeX

►

The method of isomonodromic deformations and relation formulas for the second Painlevé transcendent. Izv. Akad. Nauk SSSR Ser. Mat. 51 (4), pp. 878–892, 912 (Russian).

ⓘ

Notes:

In Russian. English translation: Math. USSR-Izv. 31(1988), no. 1, pp. 193–207

External Links:

ISSN 0373-2436, Document, MathReview (Alexander A. Pankov), ZentralBlatt

Referenced by:

§32.11(iii).

Encodings:

BibTeX

►

Quasi-linear Stokes phenomenon for the second Painlevé transcendent. Nonlinearity 16 (1), pp. 363–386.

ⓘ

External Links:

ISSN 0951-7715, Document, MathReview (Youmin Lu), ZentralBlatt

Referenced by:

§32.12(ii).

Encodings:

BibTeX

►

Connection formulae for the fourth Painlevé transcendent; Clarkson-McLeod solution. J. Phys. A 31 (17), pp. 4073–4113.

ⓘ

External Links:

ISSN 0305-4470, Document, MathReview (Yoshitsugu Takei), ZentralBlatt

Referenced by:

§32.11(v).

Encodings:

BibTeX

►

The Isomonodromic Deformation Method in the Theory of Painlevé Equations. Lecture Notes in Mathematics, Vol. 1191, Springer-Verlag, Berlin.

ⓘ

External Links:

ISBN 3-540-16483-9, MathReview (Alexander A. Pankov), ZentralBlatt

Referenced by:

§32.11(iv), §32.4(i), Profile Alexander A. Its.

Encodings:

BibTeX

…

16: 32.3 Graphics

§32.3 Graphics

►

§32.3(i) First Painlevé Equation

… ►

§32.3(ii) Second Painlevé Equation with $\alpha =0$

… ►

§32.3(iii) Fourth Painlevé Equation with $\beta =0$

… ►

►►

Figure 32.3.10: $u_k(x;\frac{5}{2})$ for $-12\le x\le 4$ with $k=\mathrm{0.24499\hspace{0.33em}2}$, $\mathrm{0.24499\hspace{0.33em}3}$. … Magnify

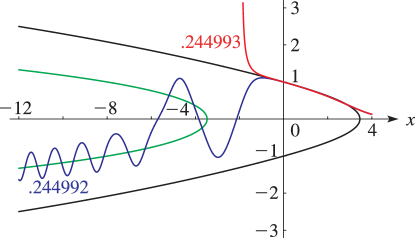17: Bibliography K

… ►

Replica field theories, Painlevé transcendents, and exact correlation functions. Phys. Rev. Lett. 89 (25), pp. (250201–1)–(250201–4).

ⓘ

External Links:

ISSN 0031-9007, Document, MathReview, ZentralBlatt

Referenced by:

§32.16.

Encodings:

BibTeX

►

Connection formulae for the first Painlevé transcendent in the complex domain. Lett. Math. Phys. 27 (4), pp. 243–252.

ⓘ

External Links:

ISSN 0377-9017, Document, MathReview (B. L. J. Braaksma), ZentralBlatt

Referenced by:

§32.11(i), §32.12(i).

Encodings:

BibTeX

… ►

Connection formulae for asymptotics of solutions of the degenerate third Painlevé equation. I. Inverse Problems 20 (4), pp. 1165–1206.

ⓘ

External Links:

ISSN 0266-5611, Document, MathReview (Shun Shimomura), ZentralBlatt

Referenced by:

§32.11(iv).

Encodings:

BibTeX

… ►

Elliptic asymptotics of the first and second Painlevé transcendents. Uspekhi Mat. Nauk 49 (1(295)), pp. 77–140 (Russian).

ⓘ

Notes:

In Russian. English translation: Russian Math. Surveys 49(1994), no. 1, pp. 81–150.

External Links:

ISSN 0042-1316, Document, MathReview (V. A. Golubeva), ZentralBlatt

Referenced by:

§32.11(i), §32.12(ii).

Encodings:

BibTeX

… ►

The Painlevé-Kowalevski and poly-Painlevé tests for integrability. Stud. Appl. Math. 86 (2), pp. 87–165.

ⓘ

External Links:

ISSN 0022-2526, MathReview (Walter Strampp), ZentralBlatt

Referenced by:

§32.2(i).

Encodings:

BibTeX

…

18: Bibliography F

… ►

On a unified approach to transformations and elementary solutions of Painlevé equations. J. Math. Phys. 23 (11), pp. 2033–2042.

ⓘ

External Links:

ISSN 0022-2488, Document, MathReview (Hélène Airault), ZentralBlatt

Referenced by:

§32.13(i), §32.7(i), §32.7(iii).

Encodings:

BibTeX

►

From continuous to discrete Painlevé equations. J. Math. Anal. Appl. 180 (2), pp. 342–360.

ⓘ

External Links:

ISSN 0022-247X, Document, MathReview (Evangelos Ifantis), ZentralBlatt

Referenced by:

§32.7(ii).

Encodings:

BibTeX

►

Discrete Painlevé equations and their appearance in quantum gravity. Comm. Math. Phys. 142 (2), pp. 313–344.

ⓘ

External Links:

ISSN 0010-3616, Link, MathReview (Nikolai A. Kostov), ZentralBlatt

Referenced by:

§32.15.

Encodings:

BibTeX

►

Continuous and Discrete Painlevé Equations. In Painlevé Transcendents: Their Asymptotics and Physical Applications, D. Levi and P. Winternitz (Eds.), NATO Adv. Sci. Inst. Ser. B Phys., Vol. 278, pp. 33–47.

ⓘ

Notes:

Proc. NATO Adv. Res. Workshop, Sainte-Adèle, Canada, 1990

External Links:

MathReview (F. Pempinelli), ZentralBlatt

Referenced by:

§32.15.

Encodings:

BibTeX

… ►

Painlevé Transcendents: The Riemann-Hilbert Approach. Mathematical Surveys and Monographs, Vol. 128, American Mathematical Society, Providence, RI.

ⓘ

External Links:

ISBN 0-8218-3651-X, MathReview Entry, ZentralBlatt

Referenced by:

§32.11(ii), §32.11(iii), §32.11(iv), §32.12(ii), §32.12(iii), §32.4(i), Profile Alexander A. Its.

Encodings:

BibTeX

…

19: Bibliography O

… ►

Studies on the Painlevé equations. III. Second and fourth Painlevé equations, $P_{\mathrm{II}}$ and $P_{\mathrm{IV}}$ . Math. Ann. 275 (2), pp. 221–255.

ⓘ

External Links:

ISSN 0025-5831, Document, MathReview (Hélène Airault), ZentralBlatt

Referenced by:

§32.10(ii), §32.10(iv), §32.6(iii), §32.6(v), §32.7(viii).

Encodings:

BibTeX

►

Studies on the Painlevé equations. I. Sixth Painlevé equation $P_{\mathrm{VI}}$ . Ann. Mat. Pura Appl. (4) 146, pp. 337–381.

ⓘ

External Links:

ISSN 0003-4622, Document, MathReview (Hélène Airault), ZentralBlatt

Referenced by:

§32.10(vi), §32.6(v), §32.7(vii), §32.7(viii).

Encodings:

BibTeX

►

Studies on the Painlevé equations. II. Fifth Painlevé equation $P_{\mathrm{V}}$ . Japan. J. Math. (N.S.) 13 (1), pp. 47–76.

ⓘ

External Links:

ISSN 0289-2316, MathReview (Hélène Airault), ZentralBlatt

Referenced by:

§32.10(v), §32.6(v), §32.7(viii).

Encodings:

BibTeX

►

Studies on the Painlevé equations. IV. Third Painlevé equation $P_{\mathrm{III}}$ . Funkcial. Ekvac. 30 (2-3), pp. 305–332.

ⓘ

External Links:

ISSN 0532-8721, FullText, MathReview (Hélène Airault), ZentralBlatt

Referenced by:

§32.10(iii), §32.2(iii), §32.6(iv), §32.7(viii).

Encodings:

BibTeX

… ►

Numerical solution of Riemann-Hilbert problems: Painlevé II. Found. Comput. Math. 11 (2), pp. 153–179.

ⓘ

External Links:

ISSN 1615-3375, Document, FullText, MathReview Entry, ZentralBlatt

Referenced by:

§32.17, §32.17.

Encodings:

BibTeX

…

20: 32.7 Bäcklund Transformations

§32.7 Bäcklund Transformations

… ►

§32.7(ii) Second Painlevé Equation

… ►

§32.7(iii) Third Painlevé Equation

… ► … ►