of Mathieu equation
(0.003 seconds)
1—10 of 54 matching pages
1: 28.2 Definitions and Basic Properties
…
►
§28.2(i) Mathieu’s Equation
… ►
28.2.1
…
►
§28.2(iii) Floquet’s Theorem and the Characteristic Exponents
… ►This is the characteristic equation of Mathieu’s equation (28.2.1). … ►§28.2(iv) Floquet Solutions
…2: 28.20 Definitions and Basic Properties
…
►
§28.20(i) Modified Mathieu’s Equation
►When is replaced by , (28.2.1) becomes the modified Mathieu’s equation: ►
28.20.1
…
►
28.20.2
.
…
►
28.20.6
,
…
3: 28.17 Stability as
§28.17 Stability as
… ► ►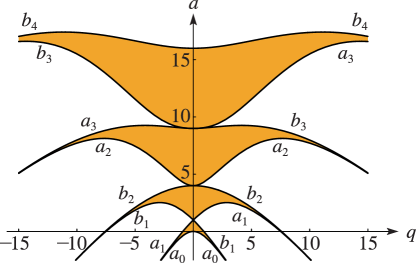 ►
►
4: 28.7 Analytic Continuation of Eigenvalues
§28.7 Analytic Continuation of Eigenvalues
… ►The normal values are simple roots of the corresponding equations (28.2.21) and (28.2.22). … ► … ►
28.7.1
…
►
28.7.4
5: 28.6 Expansions for Small
…
►
§28.6(i) Eigenvalues
… ►
28.6.14
…
►
28.6.15
►Higher coefficients in the foregoing series can be found by equating coefficients in the following continued-fraction equations:
…
►
…
6: 28.13 Graphics
…
►
§28.13(i) Eigenvalues for General
…7: 28.5 Second Solutions ,
…
►
§28.5(i) Definitions
… ► … ► … ► ►§28.5(ii) Graphics: Line Graphs of Second Solutions of Mathieu’s Equation
…8: 28.12 Definitions and Basic Properties
…
►
§28.12(i) Eigenvalues
… ►For given (or ) and , equation (28.2.16) determines an infinite discrete set of values of , denoted by , . … … ►
28.12.3
…
►They have the following pseudoperiodic and orthogonality properties:
…
