fold catastrophe

SearchAdvancedHelp

(0.001 seconds)

5 matching pages

1: 36.2 Catastrophes and Canonical Integrals

… ►

Normal Forms Associated with Canonical Integrals: Cuspoid Catastrophe with Codimension $K$

… ►Special cases: $K=1$, fold catastrophe; $K=2$, cusp catastrophe; $K=3$, swallowtail catastrophe. …

2: 36.7 Zeros

… ►

§36.7(i) Fold Canonical Integral

…

3: 36.4 Bifurcation Sets

… ►

Bifurcation (Catastrophe) Set for Cuspoids

… ►

Bifurcation (Catastrophe) Set for Umbilics

… ► $K=1$, fold bifurcation set: … ►

►►

Figure 36.4.1: Bifurcation set of cusp catastrophe. Magnify

►

►►

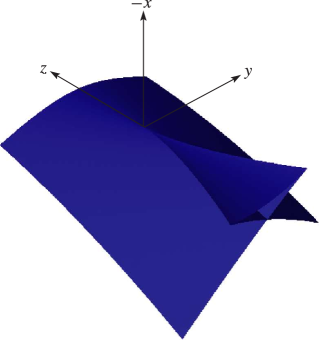
Figure 36.4.2: Bifurcation set of swallowtail catastrophe. Magnify

…

4: 36.10 Differential Equations

… ► $K=1$, fold: (36.10.1) becomes Airy’s equation (§9.2(i)) ►

36.10.3 $$\frac{{\partial }^2{\mathrm{\Psi }}_1}{{\partial x}^2}-\frac{x}{3}{\mathrm{\Psi }}_1=0.$$

ⓘ

Symbols:

${\mathrm{\Psi }}_K\left(𝐱\right)$: canonical integral function, $\frac{\partial f}{\partial x}$: partial derivative of $f$ with respect to $x$ , $\partial x$: partial differential of $x$ and $x$: real parameter

Permalink:

http://dlmf.nist.gov/36.10.E3

Encodings:

pMML, png, TeX

See also:

Annotations for §36.10(i), §36.10(i), §36.10 and Ch.36

… ► $K=1$, fold: (36.10.6) is an identity. … ►

►

…

5: 36.6 Scaling Relations

§36.6 Scaling Relations

►

Diffraction Catastrophe Scaling

►

${\mathrm{\Psi }}_K(𝐱;k)=k^{{\beta }_K}{\mathrm{\Psi }}_K\left(𝐲(k)\right),$

►

${\mathrm{\Psi }}^{(\mathrm{U})}(𝐱;k)=k^{{\beta }^{(\mathrm{U})}}{\mathrm{\Psi }}^{(\mathrm{U})}\left({𝐲}^{(\mathrm{U})}(k)\right),$

… ►

Table 36.6.1: Special cases of scaling exponents for cuspoids.

► ►►►

singularity	$K$	${\beta }_K$	${\gamma }_{1K}$	${\gamma }_{2K}$	${\gamma }_{3K}$	${\gamma }_K$
fold	1	$\frac{1}{6}$	$\frac{2}{3}$	$-$	$-$	$\frac{2}{3}$
…

►

…