Rogers–Szegő polynomials

SearchAdvancedHelp

(0.001 seconds)

21—30 of 260 matching pages

21: 24.3 Graphs

… ►

►►

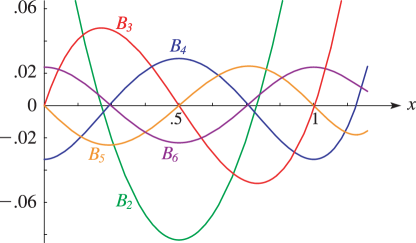
Figure 24.3.1: Bernoulli polynomials $B_n\left(x\right)$, $n=2,3,\mathrm{\dots },6$. Magnify

►

►►

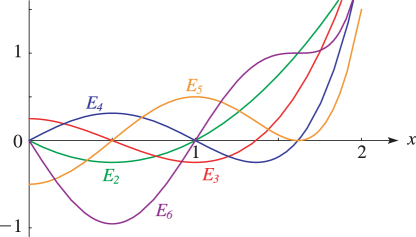
Figure 24.3.2: Euler polynomials $E_n\left(x\right)$, $n=2,3,\mathrm{\dots },6$. Magnify

22: 18.4 Graphics

… ►

►►

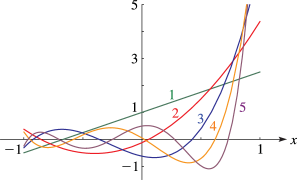
Figure 18.4.1: Jacobi polynomials $P_n^{(1.5,-0.5)}\left(x\right)$, $n=1,2,3,4,5$. Magnify

►

►►

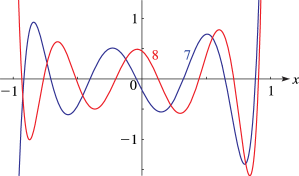
Figure 18.4.2: Jacobi polynomials $P_n^{(1.25,0.75)}\left(x\right)$, $n=7,8$. … Magnify

… ►

►►

Figure 18.4.4: Legendre polynomials $P_n\left(x\right)$, $n=1,2,3,4,5$. Magnify

►

►►

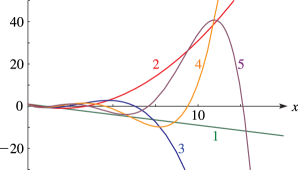Figure 18.4.5: Laguerre polynomials $L_n\left(x\right)$, $n=1,2,3,4,5$. Magnify

… ►

►►

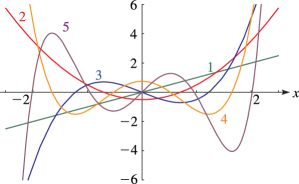Figure 18.4.7: Monic Hermite polynomials $h_n(x)=2^{-n}{H}_n\left(x\right)$, $n=1,2,3,4,5$. Magnify

…

23: 18.7 Interrelations and Limit Relations

§18.7 Interrelations and Limit Relations

… ►

Chebyshev, Ultraspherical, and Jacobi

… ►

Legendre, Ultraspherical, and Jacobi

… ►

§18.7(ii) Quadratic Transformations

… ►

§18.7(iii) Limit Relations

…

24: 18.41 Tables

… ►

§18.41(i) Polynomials

►For $P_n\left(x\right)$ ($={𝖯}_n\left(x\right)$) see §14.33. ►Abramowitz and Stegun (1964, Tables 22.4, 22.6, 22.11, and 22.13) tabulates $T_n\left(x\right)$, $U_n\left(x\right)$, $L_n\left(x\right)$, and $H_n\left(x\right)$ for $n=0(1)12$. The ranges of $x$ are $0.2(.2)1$ for $T_n\left(x\right)$ and $U_n\left(x\right)$, and $0.5,1,3,5,10$ for $L_n\left(x\right)$ and $H_n\left(x\right)$. … ►For $P_n\left(x\right)$, $L_n\left(x\right)$, and $H_n\left(x\right)$ see §3.5(v). …

25: 18.6 Symmetry, Special Values, and Limits to Monomials

… ►For Jacobi, ultraspherical, Chebyshev, Legendre, and Hermite polynomials, see Table 18.6.1. ►

Laguerre

… ►

Table 18.6.1: Classical OP’s: symmetry and special values.

► ►►

$p_n(x)$	$p_n(-x)$	$p_n(1)$	$p_{2n}(0)$	$p_{2n+1}^{\prime }(0)$
…

►

►

§18.6(ii) Limits to Monomials

… ►

18.6.4 $$\underset{\lambda \to \mathrm{\infty }}{lim}\frac{C_n^{(\lambda )}\left(x\right)}{C_n^{(\lambda )}\left(1\right)}=x^n,$$

ⓘ

Symbols:

$C_n^{(\lambda )}\left(x\right)$: ultraspherical (or Gegenbauer) polynomial, $n$: nonnegative integer and $x$: real variable

Referenced by:

§18.18(iv), §18.6(ii)

Permalink:

http://dlmf.nist.gov/18.6.E4

Encodings:

pMML, png, TeX

See also:

Annotations for §18.6(ii), §18.6 and Ch.18

…

26: 29.19 Physical Applications

§29.19 Physical Applications

… ►

§29.19(ii) Lamé Polynomials

…

27: 18.21 Hahn Class: Interrelations

§18.21 Hahn Class: Interrelations

►

§18.21(i) Dualities

… ►

§18.21(ii) Limit Relations and Special Cases

… ►

Hahn $\to$ Jacobi

… ►

Meixner $\to$ Laguerre

…

28: 18.9 Recurrence Relations and Derivatives

… ►

§18.9(iii) Derivatives

►

Jacobi

… ►

Ultraspherical

… ►

Laguerre

… ►

Hermite

…

29: 18.14 Inequalities

… ►

Legendre

… ►

Jacobi

… ►

Laguerre

… ►

Hermite

… ►

Jacobi

…

30: 18.8 Differential Equations

… ►

Table 18.8.1: Classical OP’s: differential equations $A(x)f^{\prime \prime }(x)+B(x)f^{\prime }(x)+C(x)f(x)+{\lambda }_{n}f(x)=0$.

► ►►►►►►

#	$f(x)$	$A(x)$	$B(x)$	$C(x)$	${\lambda }_n$
…
4	$C_n^{(\lambda )}\left(x\right)$	$1-x^2$	$-(2\lambda +1)x$	$0$	$n(n+2\lambda )$
…
8	$L_n^{(\alpha )}\left(x\right)$	$x$	$\alpha +1-x$	$0$	$n$
…
12	$H_n\left(x\right)$	$1$	$-2x$	$0$	$2n$
…
14	${\mathrm{𝐻𝑒}}_n\left(x\right)$	$1$	$-x$	$0$	$n$

►

…