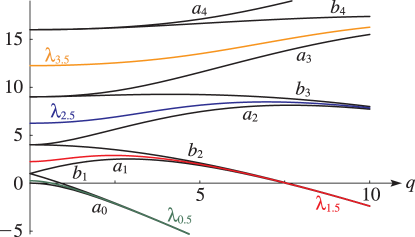
Mathieu functions

SearchAdvancedHelp

(0.006 seconds)

21—30 of 62 matching pages

21: 28.5 Second Solutions ${\mathrm{fe}}_n$, ${\mathrm{ge}}_n$

… ►

28.5.1 $${\mathrm{fe}}_n(z,q)=C_n(q)\left(z{\mathrm{ce}}_n(z,q)+f_n(z,q)\right),$$

ⓘ

Defines:

${\mathrm{fe}}_n(z,q)$: second solution, Mathieu’s equation

Symbols:

${\mathrm{ce}}_n(z,q)$: Mathieu function, $q=h^2$: parameter, $n$: integer, $z$: complex variable, $f_n(z,q)$: functions and $C_n(q)$: factor

A&S Ref:

20.3.6 (in different form)

Referenced by:

§28.5(i), §28.5(i)

Permalink:

http://dlmf.nist.gov/28.5.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §28.5(i), §28.5(i), §28.5 and Ch.28

… ►

Wronskians

►

28.5.8 $$𝒲\left\{{\mathrm{ce}}_n,{\mathrm{fe}}_n\right\}={\mathrm{ce}}_n(0,q){\mathrm{fe}}_n^{\prime }(0,q),$$

ⓘ

Symbols:

${\mathrm{ce}}_n(z,q)$: Mathieu function, ${\mathrm{fe}}_n(z,q)$: second solution, Mathieu’s equation, $𝒲$: Wronskian, $q=h^2$: parameter and $n$: integer

Permalink:

http://dlmf.nist.gov/28.5.E8

Encodings:

pMML, png, TeX

See also:

Annotations for §28.5(i), §28.5(i), §28.5 and Ch.28

… ►For further information on $C_n(q)$, $S_n(q)$, and expansions of $f_n(z,q)$, $g_n(z,q)$ in Fourier series or in series of ${\mathrm{ce}}_n$, ${\mathrm{se}}_n$ functions, see McLachlan (1947, Chapter VII) or Meixner and Schäfke (1954, §2.72). …

22: 28.10 Integral Equations

… ►

§28.10(i) Equations with Elementary Kernels

… ►

§28.10(ii) Equations with Bessel-Function Kernels

►

28.10.9 $${\int }_0^{\pi /2}{J}_0\left(2\sqrt{q({\cos }^2\tau -{\sin }^2\zeta )}\right){\mathrm{ce}}_{2n}(\tau ,q)d\tau =w_{\text{II}}(\frac{1}{2}\pi ;a_{2n}\left(q\right),q){\mathrm{ce}}_{2n}(\zeta ,q),$$

ⓘ

Symbols:

$J_{\nu }\left(z\right)$: Bessel function of the first kind, ${\mathrm{ce}}_n(z,q)$: Mathieu function, $a_n\left(q\right)$: eigenvalues of Mathieu equation, $\pi$: the ratio of the circumference of a circle to its diameter, $\cos z$: cosine function, $dx$: differential of $x$ , $\int$: integral, $\sin z$: sine function, $q=h^2$: parameter, $n$: integer and $w_{\text{II}}(z;a,q)$: fundamental solution

Permalink:

http://dlmf.nist.gov/28.10.E9

Encodings:

pMML, png, TeX

See also:

Annotations for §28.10(ii), §28.10 and Ch.28

►

28.10.10 $${\int }_0^{\pi }{J}_0\left(2\sqrt{q}(\cos \tau +\cos \zeta )\right){\mathrm{ce}}_n(\tau ,q)d\tau =w_{\text{II}}(\pi ;a_n\left(q\right),q){\mathrm{ce}}_n(\zeta ,q).$$

ⓘ

Symbols:

$J_{\nu }\left(z\right)$: Bessel function of the first kind, ${\mathrm{ce}}_n(z,q)$: Mathieu function, $a_n\left(q\right)$: eigenvalues of Mathieu equation, $\pi$: the ratio of the circumference of a circle to its diameter, $\cos z$: cosine function, $dx$: differential of $x$ , $\int$: integral, $q=h^2$: parameter, $n$: integer and $w_{\text{II}}(z;a,q)$: fundamental solution

Permalink:

http://dlmf.nist.gov/28.10.E10

Encodings:

pMML, png, TeX

See also:

Annotations for §28.10(ii), §28.10 and Ch.28

►

§28.10(iii) Further Equations

…

23: 28.15 Expansions for Small $q$

§28.15 Expansions for Small $q$

… ►

§28.15(ii) Solutions ${\mathrm{me}}_{\nu }(z,q)$

►

28.15.3 $${\mathrm{me}}_{\nu }(z,q)={\mathrm{e}}^{\mathrm{i}\nu z}-\frac{q}{4}\left(\frac{1}{\nu +1}{\mathrm{e}}^{\mathrm{i}(\nu +2)z}-\frac{1}{\nu -1}{\mathrm{e}}^{\mathrm{i}(\nu -2)z}\right)+\frac{q^2}{32}\left(\frac{1}{(\nu +1)(\nu +2)}{\mathrm{e}}^{\mathrm{i}(\nu +4)z}+\frac{1}{(\nu -1)(\nu -2)}{\mathrm{e}}^{\mathrm{i}(\nu -4)z}-\frac{2({\nu }^2+1)}{{({\nu }^2-1)}^2}{\mathrm{e}}^{\mathrm{i}\nu z}\right)+\mathrm{\cdots };$$

ⓘ

Symbols:

${\mathrm{me}}_n(z,q)$: Mathieu function, $\mathrm{e}$: base of natural logarithm, $\mathrm{i}$: imaginary unit, $q=h^2$: parameter, $z$: complex variable and $\nu$: complex parameter

A&S Ref:

20.3.17 (only two terms and without normalization)

Permalink:

http://dlmf.nist.gov/28.15.E3

Encodings:

pMML, png, TeX

See also:

Annotations for §28.15(ii), §28.15 and Ch.28

…

24: 28.9 Zeros

§28.9 Zeros

►For real $q$ each of the functions ${\mathrm{ce}}_{2n}(z,q)$, ${\mathrm{se}}_{2n+1}(z,q)$, ${\mathrm{ce}}_{2n+1}(z,q)$, and ${\mathrm{se}}_{2n+2}(z,q)$ has exactly $n$ zeros in . …

25: 31.12 Confluent Forms of Heun’s Equation

… ►This has regular singularities at $z=0$ and $1$, and an irregular singularity of rank 1 at $z=\mathrm{\infty }$. ►Mathieu functions (Chapter 28), spheroidal wave functions (Chapter 30), and Coulomb spheroidal functions (§30.12) are special cases of solutions of the confluent Heun equation. …

26: 28.26 Asymptotic Approximations for Large $q$

§28.26 Asymptotic Approximations for Large $q$

… ►

28.26.1 $${\mathrm{Mc}}_m^{(3)}(z,h)=\frac{{\mathrm{e}}^{\mathrm{i}\varphi }}{{(\pi h\cosh z)}^{1/2}}\left({\mathrm{Fc}}_m(z,h)-\mathrm{i}{\mathrm{Gc}}_m(z,h)\right),$$

ⓘ

Symbols:

$\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{e}$: base of natural logarithm, $\cosh z$: hyperbolic cosine function, $\mathrm{i}$: imaginary unit, ${\mathrm{Mc}}_n^{(j)}(z,h)$: radial Mathieu function, $m$: integer, $h$: parameter, $z$: complex variable and $\varphi$

A&S Ref:

20.9.7 (in slightly different notation)

Permalink:

http://dlmf.nist.gov/28.26.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §28.26(i), §28.26 and Ch.28

►

28.26.2 $$\mathrm{i}{\mathrm{Ms}}_{m+1}^{(3)}(z,h)=\frac{{\mathrm{e}}^{\mathrm{i}\varphi }}{{(\pi h\cosh z)}^{1/2}}\left({\mathrm{Fs}}_m(z,h)-\mathrm{i}{\mathrm{Gs}}_m(z,h)\right),$$

ⓘ

Symbols:

$\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{e}$: base of natural logarithm, $\cosh z$: hyperbolic cosine function, $\mathrm{i}$: imaginary unit, ${\mathrm{Ms}}_n^{(j)}(z,h)$: radial Mathieu function, $m$: integer, $h$: parameter, $z$: complex variable and $\varphi$

A&S Ref:

20.9.7 (in slightly different notation)

Permalink:

http://dlmf.nist.gov/28.26.E2

Encodings:

pMML, png, TeX

See also:

Annotations for §28.26(i), §28.26 and Ch.28

… ►

§28.26(ii) Uniform Approximations

…

27: 28.8 Asymptotic Expansions for Large $q$

… ►

§28.8(ii) Sips’ Expansions

… ►

§28.8(iii) Goldstein’s Expansions

… ►

Barrett’s Expansions

… ►

Dunster’s Approximations

… ►

28: 28.32 Mathematical Applications

§28.32 Mathematical Applications

►

§28.32(i) Elliptical Coordinates and an Integral Relationship

… ► … ► … ►

29: 28.24 Expansions in Series of Cross-Products of Bessel Functions or Modified Bessel Functions

§28.24 Expansions in Series of Cross-Products of Bessel Functions or Modified Bessel Functions

… ►

28.24.11 $${\mathrm{Ko}}_{2m+2}(z,h)=\sum _{\mathrm{\ell }=0}^{\mathrm{\infty }}\frac{B_{2\mathrm{\ell }+2}^{2m+2}(h^2)}{B_{2s+2}^{2m+2}(h^2)}\left(I_{\mathrm{\ell }-s}\left(h{\mathrm{e}}^{-z}\right)K_{\mathrm{\ell }+s+2}\left(h{\mathrm{e}}^z\right)-I_{\mathrm{\ell }+s+2}\left(h{\mathrm{e}}^{-z}\right)K_{\mathrm{\ell }-s}\left(h{\mathrm{e}}^z\right)\right),$$

ⓘ

Symbols:

$\mathrm{e}$: base of natural logarithm, $I_{\nu }\left(z\right)$: modified Bessel function of the first kind, $K_{\nu }\left(z\right)$: modified Bessel function of the second kind, ${\mathrm{Ko}}_n(z,h)$: modified Mathieu function, $m$: integer, $h$: parameter, $z$: complex variable and $B_m(q)$: Fourier coefficient

Permalink:

http://dlmf.nist.gov/28.24.E11

Encodings:

pMML, png, TeX

See also:

Annotations for §28.24 and Ch.28

►

28.24.12 $${\mathrm{Ke}}_{2m+1}(z,h)=\sum _{\mathrm{\ell }=0}^{\mathrm{\infty }}\frac{B_{2\mathrm{\ell }+1}^{2m+1}(h^2)}{B_{2s+1}^{2m+1}(h^2)}\left(I_{\mathrm{\ell }-s}\left(h{\mathrm{e}}^{-z}\right)K_{\mathrm{\ell }+s+1}\left(h{\mathrm{e}}^z\right)-I_{\mathrm{\ell }+s+1}\left(h{\mathrm{e}}^{-z}\right)K_{\mathrm{\ell }-s}\left(h{\mathrm{e}}^z\right)\right),$$

ⓘ

Symbols:

$\mathrm{e}$: base of natural logarithm, $I_{\nu }\left(z\right)$: modified Bessel function of the first kind, $K_{\nu }\left(z\right)$: modified Bessel function of the second kind, ${\mathrm{Ke}}_n(z,h)$: modified Mathieu function, $m$: integer, $h$: parameter, $z$: complex variable and $B_m(q)$: Fourier coefficient

Permalink:

http://dlmf.nist.gov/28.24.E12

Encodings:

pMML, png, TeX

See also:

Annotations for §28.24 and Ch.28

►

28.24.13 $${\mathrm{Ko}}_{2m+1}(z,h)=\sum _{\mathrm{\ell }=0}^{\mathrm{\infty }}\frac{A_{2\mathrm{\ell }+1}^{2m+1}(h^2)}{A_{2s+1}^{2m+1}(h^2)}\left(I_{\mathrm{\ell }-s}\left(h{\mathrm{e}}^{-z}\right)K_{\mathrm{\ell }+s+1}\left(h{\mathrm{e}}^z\right)+I_{\mathrm{\ell }+s+1}\left(h{\mathrm{e}}^{-z}\right)K_{\mathrm{\ell }-s}\left(h{\mathrm{e}}^z\right)\right).$$

ⓘ

Symbols:

$\mathrm{e}$: base of natural logarithm, $I_{\nu }\left(z\right)$: modified Bessel function of the first kind, $K_{\nu }\left(z\right)$: modified Bessel function of the second kind, ${\mathrm{Ko}}_n(z,h)$: modified Mathieu function, $m$: integer, $h$: parameter, $z$: complex variable and $A_m(q)$: Fourier coefficient

Referenced by:

§28.24, item (a)

Permalink:

http://dlmf.nist.gov/28.24.E13

Encodings:

pMML, png, TeX

See also:

Annotations for §28.24 and Ch.28

… ►For further power series of Mathieu radial functions of integer order for small parameters and improved convergence rate see Larsen et al. (2009).

30: 28.13 Graphics

§28.13 Graphics

… ►

►►

Figure 28.13.1: ${\lambda }_{\nu }\left(q\right)$ as a function of $q$ for $\nu =0.5(1)3.5$ and $a_n\left(q\right),b_n\left(q\right)$ for $n=0,1,2,3,4$ ($a$’s), $n=1,2,3,4$ ($b$’s). … Magnify

…