4 Elementary Functions Trigonometric Functions 4.14 Definitions and Periodicity 4.16 Elementary Properties

§4.15 Graphics

Referenced by:: §4.23(ii)
Permalink:: http://dlmf.nist.gov/4.15

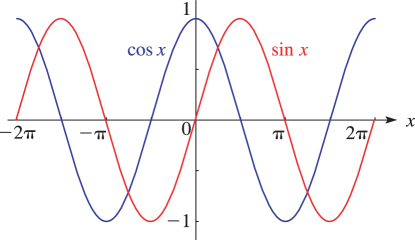
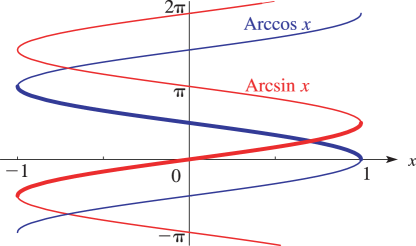
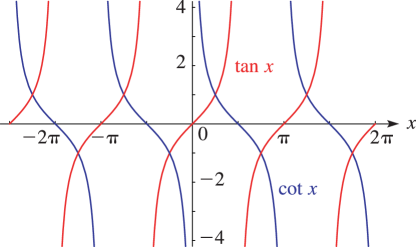
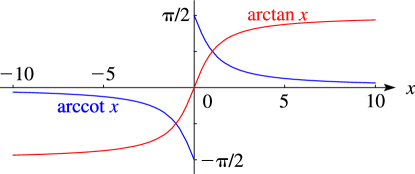
§4.15(i) Real Arguments
§4.15(ii) Complex Arguments: Conformal Maps
§4.15(iii) Complex Arguments: Surfaces

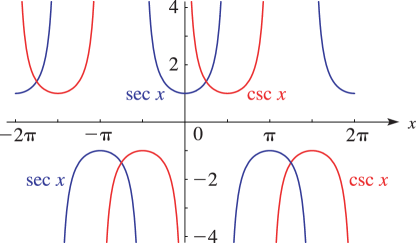
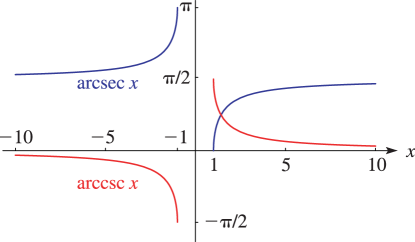
§4.15(i) Real Arguments

Notes:: These graphs were produced at NIST.
Keywords:: inverse trigonometric functions, trigonometric functions
Permalink:: http://dlmf.nist.gov/4.15.i

Figure 4.15.1: $\mathop{\sin\/}\nolimits x$ and $\mathop{\cos\/}\nolimits x$ .

Symbols:: $\mathop{\cos\/}\nolimits z$ : cosine function, $\mathop{\sin\/}\nolimits z$ : sine function and : real variable
A&S Ref:: AMS55 (Figure 4.3)
Permalink:: http://dlmf.nist.gov/4.15.F1
Encodings:: pdf, png

Figure 4.15.2: $\mathop{\mathrm{Arcsin}\/}\nolimits x$ and $\mathop{\mathrm{Arccos}\/}\nolimits x$ . Principal values are shown with thickened lines.

Symbols:: $\mathop{\mathrm{Arccos}\/}\nolimits z$ : general arccosine function, $\mathop{\mathrm{Arcsin}\/}\nolimits z$ : general arcsine function and : real variable
A&S Ref:: AMS55 (Figure 4.5)
Permalink:: http://dlmf.nist.gov/4.15.F2
Encodings:: pdf, png

Figure 4.15.3: $\mathop{\tan\/}\nolimits x$ and $\mathop{\cot\/}\nolimits x$ .

Symbols:: $\mathop{\cot\/}\nolimits z$ : cotangent function, $\mathop{\tan\/}\nolimits z$ : tangent function and : real variable
A&S Ref:: AMS55 (Figure 4.3)
Referenced by:: ¶ ‣ §3.8(ii)
Permalink:: http://dlmf.nist.gov/4.15.F3
Encodings:: pdf, png

Figure 4.15.4: $\mathop{\mathrm{arctan}\/}\nolimits x$ and $\mathop{\mathrm{arccot}\/}\nolimits x$ . Only principal values are shown. $\mathop{\mathrm{arccot}\/}\nolimits x$ is discontinuous at

Symbols:: $\mathop{\mathrm{arccot}\/}\nolimits z$ : arccotangent function, $\mathop{\mathrm{arcsec}\/}\nolimits z$ : arcsecant function, $\mathop{\mathrm{arctan}\/}\nolimits z$ : arctangent function and : real variable
A&S Ref:: AMS55 (Note that early printings of AMS55 contained errors in the graphs of $\mathop{\mathrm{arccot}\/}\nolimits$ and $\mathop{\mathrm{arcsec}\/}\nolimits$ . These errors were corrected by the 10th printing) AMS55 (Figure 4.5)
Permalink:: http://dlmf.nist.gov/4.15.F4
Encodings:: pdf, png

Figure 4.15.5: $\mathop{\csc\/}\nolimits x$ and $\mathop{\sec\/}\nolimits x$ .

Symbols:: $\mathop{\csc\/}\nolimits z$ : cosecant function, $\mathop{\sec\/}\nolimits z$ : secant function and : real variable
A&S Ref:: AMS55 (Figure 4.3)
Permalink:: http://dlmf.nist.gov/4.15.F5
Encodings:: pdf, png

Figure 4.15.6: $\mathop{\mathrm{arccsc}\/}\nolimits x$ and $\mathop{\mathrm{arcsec}\/}\nolimits x$ . Only principal values are shown. (Both functions are complex when

Symbols:: $\mathop{\mathrm{arccsc}\/}\nolimits z$ : arccosecant function, $\mathop{\mathrm{arccot}\/}\nolimits z$ : arccotangent function, $\mathop{\mathrm{arcsec}\/}\nolimits z$ : arcsecant function and : real variable
A&S Ref:: AMS55 (Note that early printings of AMS55 contained errors in the graphs of $\mathop{\mathrm{arccot}\/}\nolimits$ and $\mathop{\mathrm{arcsec}\/}\nolimits$ . These errors were corrected by the 10th printing) AMS55 (Figure 4.5)
Permalink:: http://dlmf.nist.gov/4.15.F6
Encodings:: pdf, png

§4.15(ii) Complex Arguments: Conformal Maps

Notes:: This conformal map was produced at NIST.
Permalink:: http://dlmf.nist.gov/4.15.ii

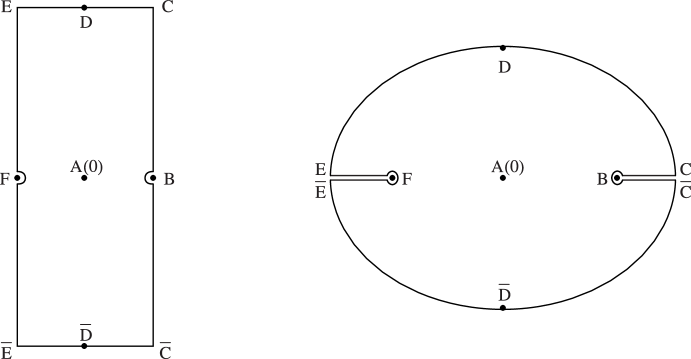
Figure 4.15.7 illustrates the conformal mapping of the strip $-\tfrac{1}{2}\pi<\realpart{z}<\tfrac{1}{2}\pi$ onto the whole -plane cut along the real axis from $-\infty$ to −1 and 1 to $\infty$ , where $w=\mathop{\sin\/}\nolimits z$ and $z=\mathop{\mathrm{arcsin}\/}\nolimits w$ (principal value). Corresponding points share the same letters, with bars signifying complex conjugates. Lines parallel to the real axis in the -plane map onto ellipses in the -plane with foci at $w=\pm 1$ , and lines parallel to the imaginary axis in the -plane map onto rectangular hyperbolas confocal with the ellipses. In the labeling of corresponding points is a real parameter that can lie anywhere in the interval $(0,\infty)$ .

(i)

-plane (ii)

-plane


A	B	C	$\mathrm{\overline{C}}$	D	$\mathrm{\overline{D}}$	E	$\mathrm{\overline{E}}$	F
0	$\tfrac{1}{2}\pi$	$\tfrac{1}{2}\pi+ir$	$\tfrac{1}{2}\pi-ir$			$-\tfrac{1}{2}\pi+ir$	$-\tfrac{1}{2}\pi-ir$	$-\tfrac{1}{2}\pi$
0	1	$\mathop{\cosh\/}\nolimits r+i0$	$\mathop{\cosh\/}\nolimits r-i0$	$i\mathop{\sinh\/}\nolimits r$	$-i\mathop{\sinh\/}\nolimits r$	$-\mathop{\cosh\/}\nolimits r+i0$	$-\mathop{\cosh\/}\nolimits r-i0$	−1

Figure 4.15.7: Conformal mapping of sine and inverse sine. $w=\mathop{\sin\/}\nolimits z$ , $z=\mathop{\mathrm{arcsin}\/}\nolimits w$ .

Symbols:: $\mathop{\cosh\/}\nolimits z$ : hyperbolic cosine function, $\mathop{\sinh\/}\nolimits z$ : hyperbolic sine function, $\mathop{\mathrm{arcsin}\/}\nolimits z$ : arcsine function, $\mathop{\sin\/}\nolimits z$ : sine function, : complex variable, : mapping and : real parameter
Keywords:: inverse trigonometric functions, trigonometric functions
Referenced by:: §4.15(ii), §4.29(ii)
Permalink:: http://dlmf.nist.gov/4.15.F7
Encodings:: pdf, png

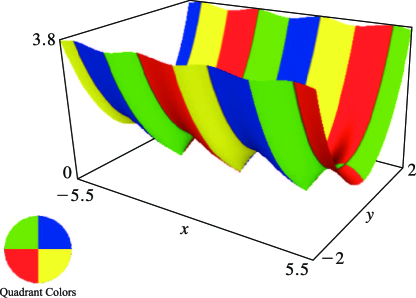
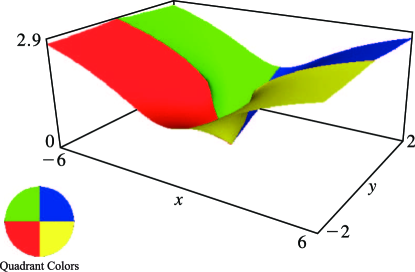
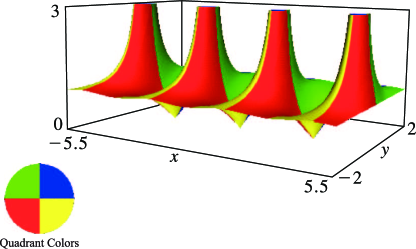
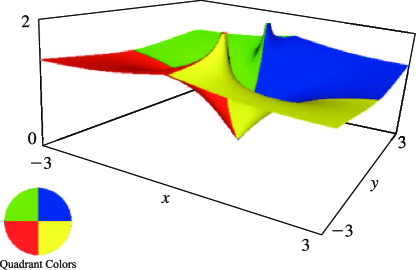
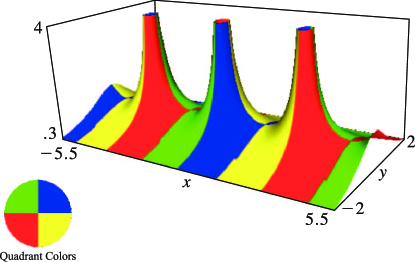
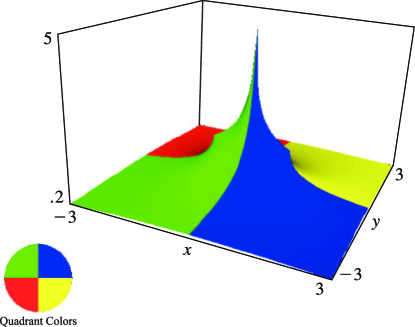
§4.15(iii) Complex Arguments: Surfaces

Notes:: These surfaces were produced at NIST.
Keywords:: inverse trigonometric functions, trigonometric functions
Referenced by:: §4.29(ii)
Permalink:: http://dlmf.nist.gov/4.15.iii

In the graphics shown in this subsection height corresponds to the absolute value of the function and color to the phase. See also About Color Map.

Visualization Help

Figure 4.15.8: $\mathop{\sin\/}\nolimits\!\left(x+iy\right)$ .

Symbols:: $\mathop{\sin\/}\nolimits z$ : sine function, : real variable and : real variable
Referenced by:: §4.15(iii)
Permalink:: http://dlmf.nist.gov/4.15.F8
Encodings:: VRML, X3D, pdf, png

Visualization Help

Figure 4.15.9: $\mathop{\mathrm{arcsin}\/}\nolimits\!\left(x+iy\right)$ (principal value). There are branch cuts along the real axis from $-\infty$ to −1 and 1 to $\infty$ .

Symbols:: $\mathop{\mathrm{arcsin}\/}\nolimits z$ : arcsine function, : real variable and : real variable
Referenced by:: §4.15(iii)
Permalink:: http://dlmf.nist.gov/4.15.F9
Encodings:: VRML, X3D, pdf, png

Visualization Help

Figure 4.15.10: $\mathop{\tan\/}\nolimits\!\left(x+iy\right)$ .

Symbols:: $\mathop{\tan\/}\nolimits z$ : tangent function, : real variable and : real variable
Referenced by:: §4.15(iii)
Permalink:: http://dlmf.nist.gov/4.15.F10
Encodings:: VRML, X3D, pdf, png

Visualization Help

Figure 4.15.11: $\mathop{\mathrm{arctan}\/}\nolimits\!\left(x+iy\right)$ (principal value). There are branch cuts along the imaginary axis from $-i\infty$ to

and

to $i\infty$ .

Symbols:: $\mathop{\mathrm{arctan}\/}\nolimits z$ : arctangent function, : real variable and : real variable
Referenced by:: §4.15(iii)
Permalink:: http://dlmf.nist.gov/4.15.F11
Encodings:: VRML, X3D, pdf, png

Visualization Help

Figure 4.15.12: $\mathop{\csc\/}\nolimits\!\left(x+iy\right)$ .

Symbols:: $\mathop{\csc\/}\nolimits z$ : cosecant function, : real variable and : real variable
Referenced by:: §4.15(iii)
Permalink:: http://dlmf.nist.gov/4.15.F12
Encodings:: VRML, X3D, pdf, png

Visualization Help

Figure 4.15.13: $\mathop{\mathrm{arccsc}\/}\nolimits\!\left(x+iy\right)$ (principal value). There is a branch cut along the real axis from −1 to 1.

Symbols:: $\mathop{\mathrm{arccsc}\/}\nolimits z$ : arccosecant function, : real variable and : real variable
Referenced by:: §4.15(iii)
Permalink:: http://dlmf.nist.gov/4.15.F13
Encodings:: VRML, X3D, pdf, png

The corresponding surfaces for $\mathop{\cos\/}\nolimits\!\left(x+iy\right)$ , $\mathop{\cot\/}\nolimits\!\left(x+iy\right)$ , and $\mathop{\sec\/}\nolimits\!\left(x+iy\right)$ are similar. In consequence of the identities

4.15.1 $\mathop{\cos\/}\nolimits\!\left(x+iy\right)=\mathop{\sin\/}\nolimits\!\left(x+% \tfrac{1}{2}\pi+iy\right),$

Symbols:: $\mathop{\cos\/}\nolimits z$ : cosine function, $\mathop{\sin\/}\nolimits z$ : sine function, : real variable and : real variable
Permalink:: http://dlmf.nist.gov/4.15.E1
Encodings:: TeX, pMML, png

4.15.2 $\mathop{\cot\/}\nolimits\!\left(x+iy\right)=-\mathop{\tan\/}\nolimits\!\left(x% +\tfrac{1}{2}\pi+iy\right),$

Symbols:: $\mathop{\cot\/}\nolimits z$ : cotangent function, $\mathop{\tan\/}\nolimits z$ : tangent function, : real variable and : real variable
Permalink:: http://dlmf.nist.gov/4.15.E2
Encodings:: TeX, pMML, png

4.15.3 $\mathop{\sec\/}\nolimits\!\left(x+iy\right)=\mathop{\csc\/}\nolimits\!\left(x+% \tfrac{1}{2}\pi+iy\right),$

Symbols:: $\mathop{\csc\/}\nolimits z$ : cosecant function, $\mathop{\sec\/}\nolimits z$ : secant function, : real variable and : real variable
Permalink:: http://dlmf.nist.gov/4.15.E3
Encodings:: TeX, pMML, png

they can be obtained by translating the surfaces shown in Figures 4.15.8, 4.15.10, 4.15.12 by $-\tfrac{1}{2}\pi$ parallel to the -axis, and adjusting the phase coloring in the case of Figure 4.15.10.

The corresponding surfaces for $\mathop{\mathrm{arccos}\/}\nolimits\!\left(x+iy\right)$ , $\mathop{\mathrm{arccot}\/}\nolimits\!\left(x+iy\right)$ , $\mathop{\mathrm{arcsec}\/}\nolimits\!\left(x+iy\right)$ can be visualized from Figures 4.15.9, 4.15.11, 4.15.13 with the aid of equations (4.23.16)–(4.23.18).