theta functions
(0.010 seconds)
1—10 of 176 matching pages
1: 20.2 Definitions and Periodic Properties
…
►
§20.2(i) Fourier Series
… ►§20.2(ii) Periodicity and Quasi-Periodicity
… ►The theta functions are quasi-periodic on the lattice: … ►§20.2(iii) Translation of the Argument by Half-Periods
… ►§20.2(iv) -Zeros
…2: 21.2 Definitions
…
►
§21.2(i) Riemann Theta Functions
… ► is also referred to as a theta function with components, a -dimensional theta function or as a genus theta function. … ►Many applications involve quotients of Riemann theta functions: the exponential factor then disappears. … ►§21.2(ii) Riemann Theta Functions with Characteristics
… ►§21.2(iii) Relation to Classical Theta Functions
…3: 21 Multidimensional Theta Functions
Chapter 21 Multidimensional Theta Functions
…4: 21.9 Integrable Equations
§21.9 Integrable Equations
… ►Typical examples of such equations are the Korteweg–de Vries equation … ► … ►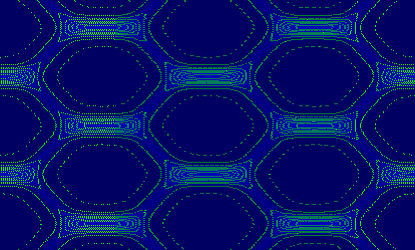 ►
►
5: 21.10 Methods of Computation
…
►
•
§21.10(i) General Riemann Theta Functions
… ►§21.10(ii) Riemann Theta Functions Associated with a Riemann Surface
… ►Deconinck and van Hoeij (2001). Here a plane algebraic curve representation of the Riemann surface is used.
6: 20.12 Mathematical Applications
§20.12 Mathematical Applications
►§20.12(i) Number Theory
… ►§20.12(ii) Uniformization and Embedding of Complex Tori
… ►Thus theta functions “uniformize” the complex torus. …7: 21.8 Abelian Functions
§21.8 Abelian Functions
… ►For every Abelian function, there is a positive integer , such that the Abelian function can be expressed as a ratio of linear combinations of products with factors of Riemann theta functions with characteristics that share a common period lattice. …8: 27.13 Functions
…
►Jacobi (1829) notes that is the coefficient of in the square of the theta function
:
►
27.13.4
.
►(In §20.2(i), is denoted by .)
…
►
27.13.6
…
►Mordell (1917) notes that is the coefficient of in the power-series expansion of the th power of the series for .
…
