stability

SearchAdvancedHelp

(0.001 seconds)

1—10 of 18 matching pages

1: 29.9 Stability

§29.9 Stability

…

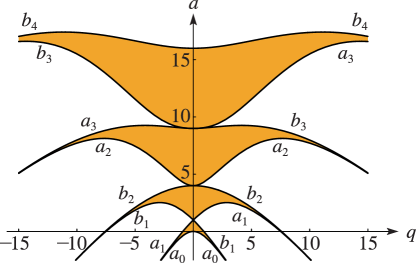
2: 28.17 Stability as $x\to \pm \mathrm{\infty }$

§28.17 Stability as $x\to \pm \mathrm{\infty }$

… ►

►►

Figure 28.17.1: Stability chart for eigenvalues of Mathieu’s equation (28.2.1). Magnify

3: Bernard Deconinck

… ►He has worked on integrable systems, algorithms for computations with Riemann surfaces, Bose-Einstein condensates, and methods to investigate the stability of solutions of nonlinear wave equations. …

4: 9.16 Physical Applications

… ►In the study of the stability of a two-dimensional viscous fluid, the flow is governed by the Orr–Sommerfeld equation (a fourth-order differential equation). …These examples of transitions to turbulence are presented in detail in Drazin and Reid (1981) with the problem of hydrodynamic stability. …

5: 11.13 Methods of Computation

… ►To insure stability the integration path must be chosen so that as we proceed along it the wanted solution grows in magnitude at least as rapidly as the complementary solutions. … ►There are similar problems to those described in §11.13(iv) concerning stability. …

6: 28.33 Physical Applications

… ►

§28.33(iii) Stability and Initial-Value Problems

►If the parameters of a physical system vary periodically with time, then the question of stability arises, for example, a mathematical pendulum whose length varies as $\cos \left(2\omega t\right)$. …

7: 10.74 Methods of Computation

… ►As described in §3.7(ii), to insure stability the integration path must be chosen in such a way that as we proceed along it the wanted solution grows in magnitude at least as fast as all other solutions of the differential equation. … ►Similarly, to maintain stability in the interval the integration direction has to be forwards in the case of $I_{\nu }\left(x\right)$ and backwards in the case of $K_{\nu }\left(x\right)$, with initial values obtained in an analogous manner to those for $J_{\nu }\left(x\right)$ and $Y_{\nu }\left(x\right)$. … ►Then $J_n\left(x\right)$ and $Y_n\left(x\right)$ can be generated by either forward or backward recurrence on $n$ when , but if $n>x$ then to maintain stability $J_n\left(x\right)$ has to be generated by backward recurrence on $n$, and $Y_n\left(x\right)$ has to be generated by forward recurrence on $n$. …

8: 9.17 Methods of Computation

… ►As described in §3.7(ii), to ensure stability the integration path must be chosen in such a way that as we proceed along it the wanted solution grows at least as fast as all other solutions of the differential equation. …

9: 3.6 Linear Difference Equations

… ►Stability can be restored, however, by backward recursion, provided that $c_n\ne 0$, $\forall n$: starting from $w_N$ and $w_{N+1}$, with $N$ large, equation (3.6.3) is applied to generate in succession $w_{N-1},w_{N-2},\mathrm{\dots },w_0$. … … ►Thus in the inhomogeneous case it may sometimes be necessary to recur backwards to achieve stability. …

10: 3.10 Continued Fractions

… ► … ►This forward algorithm achieves efficiency and stability in the computation of the convergents $C_n=A_n/B_n$, and is related to the forward series recurrence algorithm. …