parameters

SearchAdvancedHelp

(0.002 seconds)

1—10 of 386 matching pages

1: 31.13 Asymptotic Approximations

§31.13 Asymptotic Approximations

►For asymptotic approximations for the accessory parameter eigenvalues $q_m$, see Fedoryuk (1991) and Slavyanov (1996). …

2: 31.3 Basic Solutions

… ►

31.3.2 $$a\gamma c_1-q{c}_0=0,$$

ⓘ

Symbols:

$\gamma$: real or complex parameter, $a$: complex parameter, $q$: real or complex parameter and $c_j$: coefficients

Permalink:

http://dlmf.nist.gov/31.3.E2

Encodings:

pMML, png, TeX

See also:

Annotations for §31.3(i), §31.3 and Ch.31

… ►

31.3.5 $$z^{1-\gamma }H\mathrm{\ell }(a,(a\delta +ϵ)(1-\gamma )+q;\alpha +1-\gamma ,\beta +1-\gamma ,2-\gamma ,\delta ;z).$$

ⓘ

Symbols:

$H\mathrm{\ell }(a,q;\alpha ,\beta ,\gamma ,\delta ;z)$: Heun functions, $z$: complex variable, $\gamma$: real or complex parameter, $\delta$: real or complex parameter, $ϵ$: real or complex parameter, $a$: complex parameter, $q$: real or complex parameter, $\alpha$: real or complex parameter and $\beta$: real or complex parameter

Referenced by:

§31.3(iii), §31.7(ii)

Permalink:

http://dlmf.nist.gov/31.3.E5

Encodings:

pMML, png, TeX

See also:

Annotations for §31.3(i), §31.3 and Ch.31

… ►

31.3.6 $$H\mathrm{\ell }(1-a,\alpha \beta -q;\alpha ,\beta ,\delta ,\gamma ;1-z),$$

ⓘ

Symbols:

$H\mathrm{\ell }(a,q;\alpha ,\beta ,\gamma ,\delta ;z)$: Heun functions, $z$: complex variable, $\gamma$: real or complex parameter, $\delta$: real or complex parameter, $a$: complex parameter, $q$: real or complex parameter, $\alpha$: real or complex parameter and $\beta$: real or complex parameter

Permalink:

http://dlmf.nist.gov/31.3.E6

Encodings:

pMML, png, TeX

See also:

Annotations for §31.3(ii), §31.3 and Ch.31

►

31.3.7 $${(1-z)}^{1-\delta }H\mathrm{\ell }(1-a,((1-a)\gamma +ϵ)(1-\delta )+\alpha \beta -q;\alpha +1-\delta ,\beta +1-\delta ,2-\delta ,\gamma ;1-z).$$

ⓘ

Symbols:

$H\mathrm{\ell }(a,q;\alpha ,\beta ,\gamma ,\delta ;z)$: Heun functions, $z$: complex variable, $\gamma$: real or complex parameter, $\delta$: real or complex parameter, $ϵ$: real or complex parameter, $a$: complex parameter, $q$: real or complex parameter, $\alpha$: real or complex parameter and $\beta$: real or complex parameter

Permalink:

http://dlmf.nist.gov/31.3.E7

Encodings:

pMML, png, TeX

See also:

Annotations for §31.3(ii), §31.3 and Ch.31

… ►

31.3.8 $$H\mathrm{\ell }(\frac{a}{a-1},\frac{\alpha \beta a-q}{a-1};\alpha ,\beta ,ϵ,\delta ;\frac{a-z}{a-1}),$$

ⓘ

Symbols:

$H\mathrm{\ell }(a,q;\alpha ,\beta ,\gamma ,\delta ;z)$: Heun functions, $z$: complex variable, $\delta$: real or complex parameter, $ϵ$: real or complex parameter, $a$: complex parameter, $q$: real or complex parameter, $\alpha$: real or complex parameter and $\beta$: real or complex parameter

Permalink:

http://dlmf.nist.gov/31.3.E8

Encodings:

pMML, png, TeX

See also:

Annotations for §31.3(ii), §31.3 and Ch.31

…

3: 29.11 Lamé Wave Equation

… ►

29.11.1 $$\frac{d^{2}w}{{dz}^2}+(h-\nu (\nu +1)k^2{\mathrm{sn}}^2(z,k)+k^2{\omega }^2{\mathrm{sn}}^4(z,k))w=0,$$

ⓘ

Symbols:

$\mathrm{sn}(z,k)$: Jacobian elliptic function, $\frac{df}{dx}$: derivative of $f$ with respect to $x$ , $z$: complex variable, $h$: real parameter, $k$: real parameter, $\nu$: real parameter, $\omega$: parameter and $w(z)$: solution

Referenced by:

§29.11, §29.11, §29.18(ii), §29.7(ii)

Permalink:

http://dlmf.nist.gov/29.11.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §29.11 and Ch.29

►in which $\omega$ is another parameter. … ►For properties of the solutions of (29.11.1) see Arscott (1956, 1959), Arscott (1964b, Chapter X), Erdélyi et al. (1955, §16.14), Fedoryuk (1989), and Müller (1966a, b, c).

4: 31.14 General Fuchsian Equation

… ►

31.14.1 $$\frac{d^{2}w}{{dz}^2}+\left(\sum _{j=1}^N\frac{{\gamma }_j}{z-a_j}\right)\frac{dw}{dz}+\left(\sum _{j=1}^N\frac{q_j}{z-a_j}\right)w=0,$$ ${\sum }_{j=1}^N{q}_j=0$.

ⓘ

Symbols:

$\frac{df}{dx}$: derivative of $f$ with respect to $x$ , $z$: complex variable, $\gamma$: real or complex parameter, $j$: nonnegative integer, $a$: complex parameter, $q$: real or complex parameter and $N+1$: number of singularities

Referenced by:

§31.15(i)

Permalink:

http://dlmf.nist.gov/31.14.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §31.14(i), §31.14 and Ch.31

… ►

$\alpha \beta ={\displaystyle \sum _{j=1}^N}{a}_j{q}_j.$

►The three sets of parameters comprise the singularity parameters $a_j$, the exponent parameters $\alpha ,\beta ,{\gamma }_j$, and the $N-2$ free accessory parameters $q_j$. With $a_1=0$ and $a_2=1$ the total number of free parameters is $3N-3$. … ►

31.14.3 $$w(z)=\left(\prod _{j=1}^N{(z-a_j)}^{-{\gamma }_j/2}\right)W(z),$$

ⓘ

Symbols:

$z$: complex variable, $\gamma$: real or complex parameter, $j$: nonnegative integer, $a$: complex parameter, $N+1$: number of singularities and $W(z)$: solution

Permalink:

http://dlmf.nist.gov/31.14.E3

Encodings:

pMML, png, TeX

See also:

Annotations for §31.14(i), §31.14(i), §31.14 and Ch.31

…

5: 28.17 Stability as $x\to \pm \mathrm{\infty }$

§28.17 Stability as $x\to \pm \mathrm{\infty }$

►If all solutions of (28.2.1) are bounded when $x\to \pm \mathrm{\infty }$ along the real axis, then the corresponding pair of parameters $(a,q)$ is called stable. … ►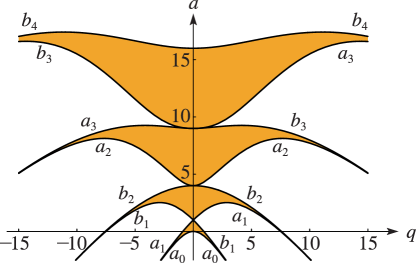

►►

Figure 28.17.1: Stability chart for eigenvalues of Mathieu’s equation (28.2.1). Magnify

6: 31.1 Special Notation

… ► ►►►►

$x$, $y$	real variables.
…
$a$	complex parameter, $|a|\ge 1,a\ne 1$.
$q,\alpha ,\beta ,\gamma ,\delta ,ϵ,\nu$	complex parameters.

… ►Sometimes the parameters are suppressed.

7: 34.8 Approximations for Large Parameters

§34.8 Approximations for Large Parameters

►For large values of the parameters in the $\mathit{3}j$, $\mathit{6}j$, and $\mathit{9}j$ symbols, different asymptotic forms are obtained depending on which parameters are large. … ►and the symbol $o\left(1\right)$ denotes a quantity that tends to zero as the parameters tend to infinity, as in §2.1(i). …

8: 28.14 Fourier Series

… ►

28.14.1 $${\mathrm{me}}_{\nu }(z,q)=\sum _{m=-\mathrm{\infty }}^{\mathrm{\infty }}{c}_{2m}^{\nu }(q){\mathrm{e}}^{\mathrm{i}(\nu +2m)z},$$

ⓘ

Symbols:

${\mathrm{me}}_n(z,q)$: Mathieu function, $\mathrm{e}$: base of natural logarithm, $\mathrm{i}$: imaginary unit, $m$: integer, $q=h^2$: parameter, $z$: complex variable, $\nu$: complex parameter and $c_{2m}(q)$: coefficients

A&S Ref:

20.3.8 (in slightly different form)

Referenced by:

§28.22(ii)

Permalink:

http://dlmf.nist.gov/28.14.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §28.14 and Ch.28

… ►

28.14.4 $$q{c}_{2m+2}-\left(a-{(\nu +2m)}^2\right)c_{2m}+q{c}_{2m-2}=0,$$ $a={\lambda }_{\nu }\left(q\right),c_{2m}=c_{2m}^{\nu }(q)$,

ⓘ

Symbols:

${\lambda }_{\nu +2n}\left(q\right)$: eigenvalues of Mathieu equation, $m$: integer, $q=h^2$: parameter, $\nu$: complex parameter, $a$: parameter and $c_{2m}(q)$: coefficients

Referenced by:

item (d), item (d)

Permalink:

http://dlmf.nist.gov/28.14.E4

Encodings:

pMML, png, TeX

See also:

Annotations for §28.14 and Ch.28

… ►

28.14.5 $$\sum _{m=-\mathrm{\infty }}^{\mathrm{\infty }}{\left(c_{2m}^{\nu }(q)\right)}^2=1;$$

ⓘ

Symbols:

$m$: integer, $q=h^2$: parameter, $\nu$: complex parameter and $c_{2m}(q)$: coefficients

Referenced by:

item (d)

Permalink:

http://dlmf.nist.gov/28.14.E5

Encodings:

pMML, png, TeX

See also:

Annotations for §28.14 and Ch.28

… ►

28.14.7 $$c_{-2m}^{-\nu }(q)=c_{2m}^{\nu }(q),$$

ⓘ

Symbols:

$m$: integer, $q=h^2$: parameter, $\nu$: complex parameter and $c_{2m}(q)$: coefficients

Permalink:

http://dlmf.nist.gov/28.14.E7

Encodings:

pMML, png, TeX

See also:

Annotations for §28.14 and Ch.28

►

28.14.8 $$c_{2m}^{\nu }(-q)={(-1)}^m{c}_{2m}^{\nu }(q).$$

ⓘ

Symbols:

$m$: integer, $q=h^2$: parameter, $\nu$: complex parameter and $c_{2m}(q)$: coefficients

Permalink:

http://dlmf.nist.gov/28.14.E8

Encodings:

pMML, png, TeX

See also:

Annotations for §28.14 and Ch.28

…

9: 15.7 Continued Fractions

… ►

15.7.1 $$\frac{𝐅(a,b;c;z)}{𝐅(a,b+1;c+1;z)}=t_0-\frac{u_{1}z}{t_1-}\frac{u_{2}z}{t_2-}\frac{u_{3}z}{t_3-}\mathrm{\cdots },$$

ⓘ

Symbols:

$𝐅(a,b;c;z)$ or $𝐅(\genfrac{}{}{0pt}{}{a,b}{c};z)$: $={}_2{}^{}𝐅_1^{}(a,b;c;z)$ Olver’s hypergeometric function, $z$: complex variable, $a$: real or complex parameter, $b$: real or complex parameter, $c$: real or complex parameter, $t_n$: coefficient and $u_n$: coefficient

Permalink:

http://dlmf.nist.gov/15.7.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §15.7 and Ch.15

… ►

15.7.3 $$\frac{𝐅(a,b;c;z)}{𝐅(a,b+1;c+1;z)}=v_0-\frac{w_1}{v_1-}\frac{w_2}{v_2-}\frac{w_3}{v_3-}\mathrm{\cdots },$$

ⓘ

Symbols:

$𝐅(a,b;c;z)$ or $𝐅(\genfrac{}{}{0pt}{}{a,b}{c};z)$: $={}_2{}^{}𝐅_1^{}(a,b;c;z)$ Olver’s hypergeometric function, $z$: complex variable, $a$: real or complex parameter, $b$: real or complex parameter, $c$: real or complex parameter, $v_n$: coefficient and $w_n$: coefficient

Permalink:

http://dlmf.nist.gov/15.7.E3

Encodings:

pMML, png, TeX

See also:

Annotations for §15.7 and Ch.15

… ►

15.7.5 $$\frac{𝐅(a,b;c;z)}{𝐅(a+1,b+1;c+1;z)}=x_0+\frac{y_1}{x_1+}\frac{y_2}{x_2+}\frac{y_3}{x_3+}\mathrm{\cdots },$$

ⓘ

Symbols:

$𝐅(a,b;c;z)$ or $𝐅(\genfrac{}{}{0pt}{}{a,b}{c};z)$: $={}_2{}^{}𝐅_1^{}(a,b;c;z)$ Olver’s hypergeometric function, $x$: real variable, $y$: real variable, $z$: complex variable, $a$: real or complex parameter, $b$: real or complex parameter and $c$: real or complex parameter

Permalink:

http://dlmf.nist.gov/15.7.E5

Encodings:

pMML, png, TeX

See also:

Annotations for §15.7 and Ch.15

…

10: 31.6 Path-Multiplicative Solutions

… ►

31.6.1 $$(s_1,s_2){\mathrm{𝐻𝑓}}_m^{\nu }(a,q_m;\alpha ,\beta ,\gamma ,\delta ;z),$$ $m=0,1,2,\mathrm{\dots }$,

ⓘ

Symbols:

$(s_1,s_2){\mathrm{𝐻𝑓}}_m(a,q_m;\alpha ,\beta ,\gamma ,\delta ;z)$: Heun functions, $z$: complex variable, $\gamma$: real or complex parameter, $\delta$: real or complex parameter, $\nu$: real or complex parameter, $m$: nonnegative integer, $a$: complex parameter, $q$: real or complex parameter, $\alpha$: real or complex parameter, $\beta$: real or complex parameter and $s_j$: parameters

Permalink:

http://dlmf.nist.gov/31.6.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §31.6 and Ch.31

…