generalized exponentials

SearchAdvancedHelp

(0.006 seconds)

1—10 of 187 matching pages

1: 8.19 Generalized Exponential Integral

§8.19 Generalized Exponential Integral

… ►

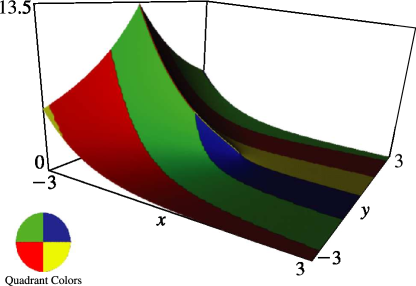
§8.19(ii) Graphics

… ►

►

►

Figure 8.19.5: $E_2\left(x+\mathrm{i}y\right)$, $-3\le x\le 3$, $-3\le y\le 3$. … Magnify 3D Help

… ►

§8.19(ix) Inequalities

… ►

§8.19(xi) Further Generalizations

…

2: 8.24 Physical Applications

§8.24 Physical Applications

… ►

§8.24(iii) Generalized Exponential Integral

… ►With more general values of $p$, $E_p\left(x\right)$ supplies fundamental auxiliary functions that are used in the computation of molecular electronic integrals in quantum chemistry (Harris (2002), Shavitt (1963)), and also wave acoustics of overlapping sound beams (Ding (2000)).

3: 4.44 Other Applications

§4.44 Other Applications

… ►For applications of generalized exponentials and generalized logarithms to computer arithmetic see §3.1(iv). …

4: 4.12 Generalized Logarithms and Exponentials

§4.12 Generalized Logarithms and Exponentials

►A generalized exponential function $\varphi (x)$ satisfies the equations … ►

4.12.2 $$\varphi (0)=0,$$

ⓘ

Symbols:

$\varphi (z)$: generalized exponential

Permalink:

http://dlmf.nist.gov/4.12.E2

Encodings:

pMML, png, TeX

See also:

Annotations for §4.12 and Ch.4

… ►

4.12.5 $$\varphi (x)=\psi (x)=x,$$ $0\le x\le 1$.

ⓘ

Symbols:

$x$: real variable, $\varphi (z)$: generalized exponential and $\psi (x)$: generalized logarithm

Permalink:

http://dlmf.nist.gov/4.12.E5

Encodings:

pMML, png, TeX

See also:

Annotations for §4.12 and Ch.4

… ►

4.12.6 $$\varphi (x)=\ln \left(x+1\right),$$ ,

ⓘ

Symbols:

$\ln z$: principal branch of logarithm function, $x$: real variable and $\varphi (z)$: generalized exponential

Permalink:

http://dlmf.nist.gov/4.12.E6

Encodings:

pMML, png, TeX

See also:

Annotations for §4.12 and Ch.4

…

5: 8.27 Approximations

… ►

§8.27(ii) Generalized Exponential Integral

►

Luke (1975, p. 103) gives Chebyshev-series expansions for $E_1\left(x\right)$ and related functions for $x\ge 5$.

…

6: 8.20 Asymptotic Expansions of $E_p\left(z\right)$

… ►

§8.20(i) Large $z$

►

8.20.1 $$E_p\left(z\right)=\frac{{\mathrm{e}}^{-z}}{z}\left(\sum _{k=0}^{n-1}{(-1)}^k\frac{{\left(p\right)}_k}{z^k}+{(-1)}^n\frac{{\left(p\right)}_n{\mathrm{e}}^z}{z^{n-1}}{E}_{n+p}\left(z\right)\right),$$ $n=1,2,3,\mathrm{\dots }$.

ⓘ

Symbols:

${\left(a\right)}_n$: Pochhammer’s symbol (or shifted factorial), $\mathrm{e}$: base of natural logarithm, $E_p\left(z\right)$: generalized exponential integral, $z$: complex variable, $p$: parameter, $k$: nonnegative integer and $n$: nonnegative integer

A&S Ref:

5.1.51

Permalink:

http://dlmf.nist.gov/8.20.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §8.20(i), §8.20 and Ch.8

… ►

8.20.2 $$E_p\left(z\right)\sim \frac{{\mathrm{e}}^{-z}}{z}\sum _{k=0}^{\mathrm{\infty }}{(-1)}^k\frac{{\left(p\right)}_k}{z^k},$$ $|\mathrm{ph}z|\le \frac{3}{2}\pi -\delta$,

ⓘ

Symbols:

${\left(a\right)}_n$: Pochhammer’s symbol (or shifted factorial), $\sim$: Poincaré asymptotic expansion, $\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{e}$: base of natural logarithm, $E_p\left(z\right)$: generalized exponential integral, $\mathrm{ph}$: phase, $z$: complex variable, $p$: parameter, $k$: nonnegative integer and $\delta$: small positive constant

Referenced by:

§8.20(i)

Permalink:

http://dlmf.nist.gov/8.20.E2

Encodings:

pMML, png, TeX

See also:

Annotations for §8.20(i), §8.20 and Ch.8

… ►Where the sectors of validity of (8.20.2) and (8.20.3) overlap the contribution of the first term on the right-hand side of (8.20.3) is exponentially small compared to the other contribution; compare §2.11(ii). … ►

§8.20(ii) Large $p$

…

7: 8.26 Tables

… ►

§8.26(iv) Generalized Exponential Integral

…

8: 8.1 Special Notation

… ►Unless otherwise indicated, primes denote derivatives with respect to the argument. ►The functions treated in this chapter are the incomplete gamma functions $\gamma (a,z)$, $\mathrm{\Gamma }(a,z)$, ${\gamma }^{\ast }(a,z)$, $P(a,z)$, and $Q(a,z)$; the incomplete beta functions ${\mathrm{B}}_x(a,b)$ and $I_x(a,b)$; the generalized exponential integral $E_p\left(z\right)$; the generalized sine and cosine integrals $\mathrm{si}(a,z)$, $\mathrm{ci}(a,z)$, $\mathrm{Si}(a,z)$, and $\mathrm{Ci}(a,z)$. …

9: 7.11 Relations to Other Functions

… ►

Incomplete Gamma Functions and Generalized Exponential Integral

… ►

7.11.3 $$\mathrm{erfc}z=\frac{z}{\sqrt{\pi }}{E}_{\frac{1}{2}}\left(z^2\right).$$

ⓘ

Symbols:

$\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{erfc}z$: complementary error function, $E_p\left(z\right)$: generalized exponential integral and $z$: complex variable

Referenced by:

§7.12(i)

Permalink:

http://dlmf.nist.gov/7.11.E3

Encodings:

pMML, png, TeX

See also:

Annotations for §7.11, §7.11 and Ch.7

…

10: 8.22 Mathematical Applications

§8.22 Mathematical Applications

… ►

8.22.1 $$F_p\left(z\right)=\frac{\mathrm{\Gamma }\left(p\right)}{2\pi }{z}^{1-p}{E}_p\left(z\right)=\frac{\mathrm{\Gamma }\left(p\right)}{2\pi }\mathrm{\Gamma }(1-p,z),$$

ⓘ

Symbols:

$\mathrm{\Gamma }\left(z\right)$: gamma function, $\pi$: the ratio of the circumference of a circle to its diameter, $E_p\left(z\right)$: generalized exponential integral, $\mathrm{\Gamma }(a,z)$: incomplete gamma function, $F_p\left(z\right)$: terminant function, $z$: complex variable and $p$: parameter

Permalink:

http://dlmf.nist.gov/8.22.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §8.22(i), §8.22 and Ch.8

…