complementary error function

SearchAdvancedHelp

(0.008 seconds)

1—10 of 46 matching pages

1: 7.18 Repeated Integrals of the Complementary Error Function

§7.18 Repeated Integrals of the Complementary Error Function

… ►

${\mathrm{i}}^{-1}\mathrm{erfc}\left(z\right)={\displaystyle \frac{2}{\sqrt{\pi }}}{\mathrm{e}}^{-z^2},$

►

${\mathrm{i}}^0\mathrm{erfc}\left(z\right)=\mathrm{erfc}z,$

… ►

7.18.2 $${\mathrm{i}}^n\mathrm{erfc}\left(z\right)={\int }_z^{\mathrm{\infty }}{\mathrm{i}}^{n-1}\mathrm{erfc}\left(t\right)dt=\frac{2}{\sqrt{\pi }}{\int }_z^{\mathrm{\infty }}\frac{{(t-z)}^n}{n!}{\mathrm{e}}^{-t^2}dt.$$

ⓘ

Defines:

${\mathrm{i}}^n\mathrm{erfc}\left(z\right)$: repeated integrals of the complementary error function

Symbols:

$\pi$: the ratio of the circumference of a circle to its diameter, $dx$: differential of $x$ , $\mathrm{e}$: base of natural logarithm, $!$: factorial (as in $n!$), $\int$: integral, $z$: complex variable and $n$: nonnegative integer

A&S Ref:

7.2.1 (in modified form) 7.2.3

Permalink:

http://dlmf.nist.gov/7.18.E2

Encodings:

pMML, png, TeX

See also:

Annotations for §7.18(i), §7.18 and Ch.7

… ►

7.18.7 $${\mathrm{i}}^n\mathrm{erfc}\left(z\right)=-\frac{z}{n}{\mathrm{i}}^{n-1}\mathrm{erfc}\left(z\right)+\frac{1}{2n}{\mathrm{i}}^{n-2}\mathrm{erfc}\left(z\right),$$ $n=1,2,3,\mathrm{\dots }$.

ⓘ

Symbols:

${\mathrm{i}}^n\mathrm{erfc}\left(z\right)$: repeated integrals of the complementary error function, $z$: complex variable and $n$: nonnegative integer

A&S Ref:

7.2.5

Referenced by:

§7.22(iii)

Permalink:

http://dlmf.nist.gov/7.18.E7

Encodings:

pMML, png, TeX

See also:

Annotations for §7.18(iii), §7.18 and Ch.7

…

2: 7.25 Software

… ►

§7.25(ii) $\mathrm{erf}x$, $\mathrm{erfc}x$, ${\mathrm{i}}^n\mathrm{erfc}\left(x\right)$, $x\in ℝ$

… ►

§7.25(iii) $\mathrm{erf}z$, $\mathrm{erfc}z$, $w\left(z\right)$, $z\in ℂ$

…

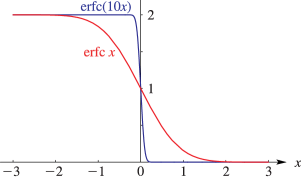
3: 7.3 Graphics

… ►

►►

Figure 7.3.1: Complementary error functions $\mathrm{erfc}x$ and $\mathrm{erfc}\left(10x\right)$, $-3\le x\le 3$. Magnify

… ►

►

►

Figure 7.3.6: $|\mathrm{erfc}\left(x+\mathrm{i}y\right)|$, $-3\le x\le 3$, $-3\le y\le 3$. … Magnify 3D Help

4: 7.22 Methods of Computation

… ►

§7.22(iii) Repeated Integrals of the Complementary Error Function

►The recursion scheme given by (7.18.1) and (7.18.7) can be used for computing ${\mathrm{i}}^n\mathrm{erfc}\left(x\right)$. … ►The computation of these functions can be based on algorithms for the complementary error function with complex argument; compare (7.19.3). …

5: 7.1 Special Notation

… ►The main functions treated in this chapter are the error function $\mathrm{erf}z$; the complementary error functions $\mathrm{erfc}z$ and $w\left(z\right)$; Dawson’s integral $F\left(z\right)$; the Fresnel integrals $ℱ\left(z\right)$, $C\left(z\right)$, and $S\left(z\right)$; the Goodwin–Staton integral $G\left(z\right)$; the repeated integrals of the complementary error function ${\mathrm{i}}^n\mathrm{erfc}\left(z\right)$; the Voigt functions $𝖴(x,t)$ and $𝖵(x,t)$. ►Alternative notations are $Q(z)=\frac{1}{2}\mathrm{erfc}\left(z/\sqrt{2}\right)$, $P(z)=\mathrm{\Phi }(z)=\frac{1}{2}\mathrm{erfc}\left(-z/\sqrt{2}\right)$, $\mathrm{Erf}z=\frac{1}{2}\sqrt{\pi }\mathrm{erf}z$, $\mathrm{Erfi}z={\mathrm{e}}^{z^2}F\left(z\right)$, $C_1(z)=C\left(\sqrt{2/\pi }z\right)$, $S_1(z)=S\left(\sqrt{2/\pi }z\right)$, $C_2(z)=C\left(\sqrt{2z/\pi }\right)$, $S_2(z)=S\left(\sqrt{2z/\pi }\right)$. … ►

6: 7.24 Approximations

… ►

Hastings (1955) gives several minimax polynomial and rational approximations for $\mathrm{erf}x$, $\mathrm{erfc}x$ and the auxiliary functions $\mathrm{f}\left(x\right)$ and $\mathrm{g}\left(x\right)$.

►

Cody (1969) provides minimax rational approximations for $\mathrm{erf}x$ and $\mathrm{erfc}x$. The maximum relative precision is about 20S.

… ►

Schonfelder (1978) gives coefficients of Chebyshev expansions for $x^{-1}\mathrm{erf}x$ on $0\le x\le 2$, for $x{\mathrm{e}}^{x^2}\mathrm{erfc}x$ on $[2,\mathrm{\infty })$, and for ${\mathrm{e}}^{x^2}\mathrm{erfc}x$ on $[0,\mathrm{\infty })$ (30D).

►

Shepherd and Laframboise (1981) gives coefficients of Chebyshev series for $(1+2x){\mathrm{e}}^{x^2}\mathrm{erfc}x$ on $(0,\mathrm{\infty })$ (22D).

… ►

Luke (1969b, vol. 2, pp. 422–435) gives main diagonal Padé approximations for $F\left(z\right)$, $\mathrm{erf}z$, $\mathrm{erfc}z$, $C\left(z\right)$, and $S\left(z\right)$; approximate errors are given for a selection of $z$-values.

7: 7.9 Continued Fractions

… ►

7.9.1 $$\sqrt{\pi }{\mathrm{e}}^{z^2}\mathrm{erfc}z=\frac{z}{z^2+}\frac{\frac{1}{2}}{1+}\frac{1}{z^2+}\frac{\frac{3}{2}}{1+}\frac{2}{z^2+}\mathrm{\cdots },$$ $\mathrm{\Re }z>0$,

ⓘ

Symbols:

$\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{erfc}z$: complementary error function, $\mathrm{e}$: base of natural logarithm, $\mathrm{\Re }$: real part and $z$: complex variable

A&S Ref:

7.1.14 (in different form)

Referenced by:

§7.9

Permalink:

http://dlmf.nist.gov/7.9.E1

Encodings:

pMML, png, TeX

See also:

Annotations for §7.9 and Ch.7

►

7.9.2 $$\sqrt{\pi }{\mathrm{e}}^{z^2}\mathrm{erfc}z=\frac{2z}{2z^2+1-}\frac{1\cdot 2}{2z^2+5-}\frac{3\cdot 4}{2z^2+9-}\mathrm{\cdots },$$ $\mathrm{\Re }z>0$,

ⓘ

Symbols:

$\pi$: the ratio of the circumference of a circle to its diameter, $\mathrm{erfc}z$: complementary error function, $\mathrm{e}$: base of natural logarithm, $\mathrm{\Re }$: real part and $z$: complex variable

Referenced by:

§7.9

Permalink:

http://dlmf.nist.gov/7.9.E2

Encodings:

pMML, png, TeX

See also:

Annotations for §7.9 and Ch.7

…

8: 7.17 Inverse Error Functions

… ►The inverses of the functions $x=\mathrm{erf}y$, $x=\mathrm{erfc}y$, $y\in ℝ$, are denoted by … ►

$y=\mathrm{inverfc}x,$

… ►

§7.17(iii) Asymptotic Expansion of $\mathrm{inverfc}x$ for Small $x$

… ►

7.17.3 $$\mathrm{inverfc}x\sim u^{-1/2}+a_2{u}^{3/2}+a_3{u}^{5/2}+a_4{u}^{7/2}+\mathrm{\cdots },$$

ⓘ

Symbols:

$\sim$: Poincaré asymptotic expansion, $\mathrm{inverfc}x$: inverse complementary error function, $x$: real variable, $a_{\mathrm{i}}$: coefficients and $u$: expansion variable

Referenced by:

§7.17(iii), §7.17(iii)

Permalink:

http://dlmf.nist.gov/7.17.E3

Encodings:

pMML, png, TeX

See also:

Annotations for §7.17(iii), §7.17 and Ch.7

…

9: 7.21 Physical Applications

§7.21 Physical Applications

… ►Carslaw and Jaeger (1959) gives many applications and points out the importance of the repeated integrals of the complementary error function ${\mathrm{i}}^n\mathrm{erfc}\left(z\right)$. …

10: 7.23 Tables

… ►

Abramowitz and Stegun (1964, Chapter 7) includes $\mathrm{erf}x$, $(2/\sqrt{\pi }){\mathrm{e}}^{-x^2}$, $x\in [0,2]$, 10D; $(2/\sqrt{\pi }){\mathrm{e}}^{-x^2}$, $x\in [2,10]$, 8S; $x{\mathrm{e}}^{x^2}\mathrm{erfc}x$, $x^{-2}\in [0,0.25]$, 7D; $2^n\mathrm{\Gamma }\left(\frac{1}{2}n+1\right){\mathrm{i}}^n\mathrm{erfc}\left(x\right)$, $n=1(1)6,10,11$, $x\in [0,5]$, 6S; $F\left(x\right)$, $x\in [0,2]$, 10D; $xF\left(x\right)$, $x^{-2}\in [0,0.25]$, 9D; $C\left(x\right)$, $S\left(x\right)$, $x\in [0,5]$, 7D; $\mathrm{f}\left(x\right)$, $\mathrm{g}\left(x\right)$, $x\in [0,1]$, $x^{-1}\in [0,1]$, 15D.

… ►

Zhang and Jin (1996, pp. 637, 639) includes $(2/\sqrt{\pi }){\mathrm{e}}^{-x^2}$, $\mathrm{erf}x$, $x=0(.02)1(.04)3$, 8D; $C\left(x\right)$, $S\left(x\right)$, $x=0(.2)10(2)100(100)500$, 8D.

… ►

Fettis et al. (1973) gives the first 100 zeros of $\mathrm{erf}z$ and $w\left(z\right)$ (the table on page 406 of this reference is for $w\left(z\right)$, not for $\mathrm{erfc}z$), 11S.

…