Painlevé transcendents

SearchAdvancedHelp

(0.002 seconds)

11—20 of 33 matching pages

11: Peter A. Clarkson

… ►

32 Painlevé Transcendents

…

12: Mark J. Ablowitz

… ►ODEs with the Painlevé property contain the well-known Painlevé equations which are special second order scalar equations; their solutions are often called Painlevé transcendents. …

13: 32.2 Differential Equations

… ►

§32.2(i) Introduction

►The six Painlevé equations ${\text{P}}_{\text{I}}$–${\text{P}}_{\text{VI}}$ are as follows: … ►The solutions of ${\text{P}}_{\text{I}}$–${\text{P}}_{\text{VI}}$ are called the Painlevé transcendents. The six equations are sometimes referred to as the Painlevé transcendents, but in this chapter this term will be used only for their solutions. …

14: 32.4 Isomonodromy Problems

… ►Suppose …

15: Bibliography I

… ►

The method of isomonodromic deformations and relation formulas for the second Painlevé transcendent. Izv. Akad. Nauk SSSR Ser. Mat. 51 (4), pp. 878–892, 912 (Russian).

ⓘ

Notes:

In Russian. English translation: Math. USSR-Izv. 31(1988), no. 1, pp. 193–207

External Links:

ISSN 0373-2436, Document, MathReview (Alexander A. Pankov), ZentralBlatt

Referenced by:

§32.11(iii).

Encodings:

BibTeX

►

Quasi-linear Stokes phenomenon for the second Painlevé transcendent. Nonlinearity 16 (1), pp. 363–386.

ⓘ

External Links:

ISSN 0951-7715, Document, MathReview (Youmin Lu), ZentralBlatt

Referenced by:

§32.12(ii).

Encodings:

BibTeX

►

Connection formulae for the fourth Painlevé transcendent; Clarkson-McLeod solution. J. Phys. A 31 (17), pp. 4073–4113.

ⓘ

External Links:

ISSN 0305-4470, Document, MathReview (Yoshitsugu Takei), ZentralBlatt

Referenced by:

§32.11(v).

Encodings:

BibTeX

…

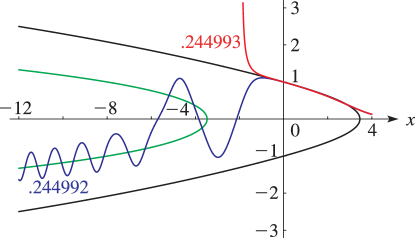
16: 32.3 Graphics

§32.3 Graphics

… ►

►►

Figure 32.3.10: $u_k(x;\frac{5}{2})$ for $-12\le x\le 4$ with $k=\mathrm{0.24499\hspace{0.33em}2}$, $\mathrm{0.24499\hspace{0.33em}3}$. … Magnify

17: 32.7 Bäcklund Transformations

§32.7 Bäcklund Transformations

… ►With the exception of ${\text{P}}_{\text{I}}$, a Bäcklund transformation relates a Painlevé transcendent of one type either to another of the same type but with different values of the parameters, or to another type. … ►

18: 32.6 Hamiltonian Structure

§32.6 Hamiltonian Structure

… ►

19: 32.11 Asymptotic Approximations for Real Variables

§32.11 Asymptotic Approximations for Real Variables

… ► …

20: Bibliography K

… ►

Replica field theories, Painlevé transcendents, and exact correlation functions. Phys. Rev. Lett. 89 (25), pp. (250201–1)–(250201–4).

ⓘ

External Links:

ISSN 0031-9007, Document, MathReview, ZentralBlatt

Referenced by:

§32.16.

Encodings:

BibTeX

►

Connection formulae for the first Painlevé transcendent in the complex domain. Lett. Math. Phys. 27 (4), pp. 243–252.

ⓘ

External Links:

ISSN 0377-9017, Document, MathReview (B. L. J. Braaksma), ZentralBlatt

Referenced by:

§32.11(i), §32.12(i).

Encodings:

BibTeX

… ►

Elliptic asymptotics of the first and second Painlevé transcendents. Uspekhi Mat. Nauk 49 (1(295)), pp. 77–140 (Russian).

ⓘ

Notes:

In Russian. English translation: Russian Math. Surveys 49(1994), no. 1, pp. 81–150.

External Links:

ISSN 0042-1316, Document, MathReview (V. A. Golubeva), ZentralBlatt

Referenced by:

§32.11(i), §32.12(ii).

Encodings:

BibTeX

…